 Fig.1
Fig.1While it is probable that most of those reading this article are doing so because they already know what a Saros Series is and are simply hoping to gain more knowledge about them, I cannot assume this is generally true. I shall therefore start with a quick description, and also run through some aspects of "Eclipse Theory" so that subsequent sections hopefully make a bit more sense. "The cognoscenti" will therefore have to bear with me for a while!
At the most basic level, an Eclipse Series is simply a series of eclipses (either solar or lunar) which all have the same time interval between them. There are, in fact, many such series but most of them are quite short-lived i.e. there are only a few eclipses in the series before it dies out. A Saros Series, by contrast, runs for many tens of eclipses because of the close arithmetical correspondence between the astronomical factors which produce it.
The fundamental ideas behind Saros (and other) Series go right back into antiquity, as both Babylonian and Chinese astronomers were aware from the records they kept (based on reports brought back to them by envoys to "foreign lands") that eclipses tended to run in cycles, and that an 18-year cycle was particularly common i.e. there would generally have been an eclipse 18, 36, 54 (etc.) years before any given eclipse. This does not mean that eclipses were always 18 years apart of course, as several cycles were operative at any one time. Knowledge of eclipse cycles could be very useful, as predicting eclipses (which were thought to be bad omens) was an important part of a court astronomer's job: get it wrong and you would lose more than your salary!
So that's what a Saros Series is - a series of eclipses 18 years apart. Now on to a bit of theory - necessary to explain the basic arithmetic behind the 18-year interval.
An eclipse of the Sun or Moon is only possible if the three bodies involved are lying in a straight line - in the order Sun-Moon-Earth for a solar eclipse and Sun-Earth-Moon for a lunar. As I show in my article on Eclipse Limits, it does not have to be a "geometrically perfect" straight line (mainly because of the significant physical size of the Earth) but a reasonably close alignment is required nonetheless. Taking the case of a solar eclipse, the only time the Moon can lie between the Sun and the Earth is when it is New. However, because the plane of the Moon's orbit is slightly inclined with respect to that of the Earth, simply being New is not enough: the Moon must also sit at one of the two points where the planes intersect (called nodes), to ensure it can be directly on the Sun-Earth line and not above or below it. Even this is not enough though, because the line joining the nodes does not always point towards the Sun - it is almost fixed in space. "Almost" because, due to the effect of the gravitational attraction of the Sun, the line does actually move its "direction of point" round in an entire circle every 18.61 years. This rotation is in the opposite direction to that in which the Earth orbits the Sun and so instead of one node or the other being exactly between Sun and Earth every 6 months the period is in fact 173.31 days. The argument is exactly the same for lunar eclipses, with the slight differences that the Moon must be Full rather than New and it must sit at the node which is on the far side of the Earth from the Sun rather than the near side.
Because of the above constraints, once an eclipse has happened another similar one will not occur until a whole number of lunations (new-Moon-to-new-Moon lunar months), node-crossing periods and node-alignment intervals have passed, thus ensuring that the Moon is back to where it started for each of the three criteria. However, because the Moon's orbit is somewhat eccentric rather than exactly circular, this next eclipse will almost certainly not be identical to the one before: if the Moon is further away from the Earth (and therefore apparently smaller) the next eclipse might be annular rather than total, for example. To ensure the maximum similarity we must therefore also factor in the time taken to go from one perigee (the point at which the Moon is nearest to the Earth) to the next. These relationships constitute the underlying arithmetic of Saros series.
We now know that the Saros period is the name given to the interval of a little over 18 years after which, as explained above, the circumstances of an eclipse (solar or lunar) repeat almost exactly. It is equal to 223 lunations, each of mean duration 29.53 days: this interval is also very closely equal to 242 node-crossing periods (of 27.21 days), 38 node-alignment intervals or "eclipse seasons" (of 173.31 days), and 238 perigee-to-perigee months (of 27.55 days). A Saros Series consists of a set of eclipses each of which is one Saros period from the next. For example, the famous total solar eclipses of 11th August 1999 (the "Cornish") and 21st August 2017 (the "American") are members of the same Saros series.
The story of how the 18-year interval (actually 18 years and 10 or 11 days) became called a Saros is a complicated one, so I have placed it on a separate page - click here to read about it.
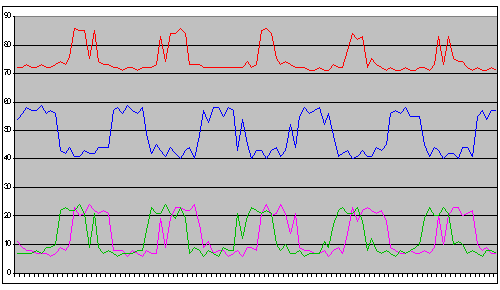
Although the correspondence between the all the periods mentioned above is very close, it is not exact. Using accurate figures, the "lunations" version of the Saros period comes out as 6585.321 days: the difference between this and the "eclipse seasons" version is 0.461 days. Successive eclipses do not, therefore, precisely repeat their circumstances. A Saros series of solar eclipses starts with a number of partial eclipses, has a larger number of total or annular eclipses in the middle of its "life", and ends with a further number of partials. The number of eclipses in each phase of a series is highly variable though, a fact which first came to my attention as a result of reading 'Mathematical Astronomy Morsels III' by Jean Meeus. In this book Meeus gives a diagram of the numbers of opening partial, non-partial (i.e. total and annular) and closing partial eclipses for each solar Saros series with a start date from 1806BC to 2134AD. The figure below gives a version of this diagram that I constructed for series from about 3000BC to 250AD as this interval shows the situation even more clearly.
 Fig.1
Fig.1
The top graph shows the overall number of eclipses, the middle one the number of non-partials (totals and annulars) and the lower ones the number of opening [pink] and closing [green] partials for each series between these dates.
The most obvious question we must answer is, given the relative simplicity of the above description of the Saros, based as it is on simple multiples of basic orbital periods, why is there a variability in these graphs at all? (let alone such a dramatic one). To then touch on just a few of the additional puzzles illustrated by the figure, it is clear that the total number of eclipses in a Saros series tends to have one of only two values, with a rapid switch between them; that there are far fewer series with large totals than with small totals, and that large values occur with a definite regularity. Many more questions will no doubt suggest themselves to the observer but, to quote Meeus "We fear, however, that there is no simple explanation for these variations in the composition of the successive Saros series." - how right he was!
Before plunging in to explore these conundrums, we must first tackle a rather basic issue. Clearly, Saros series cannot be listed in order until an order has actually been established i.e. until all series have been numbered. Our first attempt at doing this might be to find an eclipse which is the first of a particular series (because there is no eclipse one Saros period earlier) and call this the first member of series 1. The next eclipse after this would be a member of series 2, the next after that a member of series 3 and so on until we get to the eclipse which is one Saros period after the first one, which becomes the second member of series 1. Unfortunately, this rule only leads to confusion. For example, the eclipse we listed as a member of series 2 is highly unlikely to be the first member of that series and so series 2 must have started before series 1! Likewise, series 3 must also have started before series 1, but we cannot be certain whether this was after series 2 or before it!! The proposed scheme thus results in series numbers which bear no relation to the chronology of the eclipses. Also, because eclipses may be 1, 5 or 6 lunar months apart, even if the numbering scheme were to be logical there would be no simple relationship between the resulting series.
The solution was found by Prof. Dr. George van den Bergh, using the catalogue of eclipses compiled by the astronomer Oppolzer in 1887. He concentrated on the series, not the eclipses, and found that if he grouped all the eclipses into series where the dates of successive members of the series differed by one Saros period and then placed the series as columns on a grid, the columns could be arranged in a regular pattern if eclipses on the same row in adjacent columns were separated by a period of 358 lunar months (just less than 29yrs) which he called the Inex. Because of its relationship to the Saros and Inex periods, van den Bergh called the pattern produced by his arrangement the "Saros-Inex Panorama".
Van den Bergh numbered the columns of the Panorama grid (and hence the Saros series) sequentially from left to right. In the case of solar eclipses, he gave the value 0 to the column starting with the eclipse which began the first new series of the second millennium BC (whose dates he derived by extrapolating those in Oppolzer's catalogue). Solar Saros series 0 thus begins on 23rd May 2956 BC. However, for reasons that aren't entirely clear, lunar Saros series 0 does not begin on a notable date but rather on 1st March 2654 BC!
Although van den Bergh's method solves many problems of numbering, it introduces others such as the fact that successive eclipses do not have successive Saros series numbers. To further explore the numbering of Saros series, click here.
Since the time the original Panorama was defined it has been extended to include eclipses before 3000BC - the series containing these eclipses have negative series numbers. This means that the lunar Saros series starting closest to the beginning of the second millennium BC (corresponding to solar series 0) is in fact series -8 (7th June 2994 BC). Fig.2 shows a section from the solar Panorama. Note the regular "sawtooth" appearance of the top and bottom edges and the fact that the "teeth" of the two edges are not aligned - this is more easily seen in the expanded view given by Fig.2a where I've drawn lines to show this.
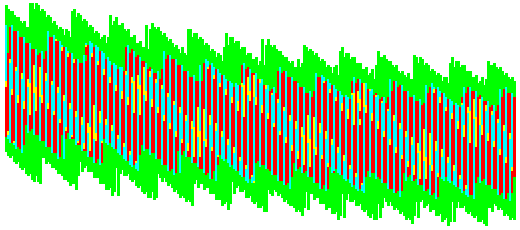 Fig.2 The green areas represent partial eclipses, the blue ones total eclipses and the red ones annulars. The yellow areas are "hybrid" eclipses - these happen when the Moon-Earth distance is right on the borderline between total and annular eclipses. The eclipse type thus changes from annular to total and then back again along the eclipse track due to the variation in distance caused by the curvature of the Earth. | 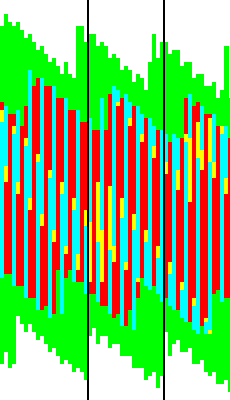 Fig.2a |
While it is clear that the way of arranging eclipses derived by van den Bergh does indeed give a regular pattern, the Panorama diagram almost suggests more questions than it answers! For example - why does it have a sawtooth appearance? Why are the teeth not aligned? Why do the types of non-partial eclipse vary in the way they do? Why is the pattern not more regular than it actually is? The answers to these additional questions (and many more!) will be given as this article develops.
Before moving on, however, I think it would be helpful to say a few more things about the Inex.
The name "Inex" comes from its association with the in-coming and ex-iting of eclipse series. Though it is but one of a large number of periods that can be derived from various combinations of new Moon and node-crossing months, it is actually a very precise one. Made up of 358 "new Moon" months, its mean length is 10571.95days, or 29 calendar years minus 20 or 21 days (depending on how many leap years are spanned). To a error of only 0.003days this is also 388.5 "node crossing" months - the extra half just means that eclipses separated by one Inex happen at opposite nodes. This is confirmed by the fact that an Inex is 61 "node line-up" periods (or eclipse seasons) long: successive eclipse seasons happen at opposite nodes so an odd number of them will result in a swap of nodes overall. This alternation of nodes means that eclipses in Saros series having an even number occur at the Moon's descending node while those in series with an odd number occur at the ascending node. All eclipses of a given series are at the same node, as the Saros period is a whole number of node-crossing months.
The precision of the agreement of the Inex periodicities (12 times better than for the Saros) means that the "geometric" circumstances of eclipses separated by one Inex are almost precisely the same. However! The Inex has a most important downside. A Saros period is not only close to a whole number of new Moon ["synodic"] and node crossing ["draconic"] months but also to a whole number of perigee to perigee ["anomalistic"] months. All three cycles thus keep very closely in step from one eclipse in the series to the next. Conversely, although an Inex period is very close to a whole number of synodic months and to a whole number plus a half of draconic months, it is only equal to a whole number plus two-thirds of anomalistic months. This means that the new Moon and apogee/perigee cycles will only be in step once every three Inexes. Given that the Moon-Earth distance is the major factor in determining whether an eclipse will be total or annular, this means that the relative numbers of these two types of "full" eclipse in a given Saros series varies quite considerably. There are also other surprisingly extensive consequences of this situation which conspire to complicate many aspects of the development of Saros series - I shall analyse these much more fully in due course.
Any glance at a list of Saros series (such as those on the NASA website) will show that not only are series numbered in the slightly strange way described above but a) successive Saros series do not actually run in order of their start dates, and b) the time difference between their start dates is highly irregular. It is thus by no means clear that anything about them should be periodic in the first place! We can gain some insight into this simply by considering the dates of the first eclipse of each series, when two very interesting relationships become apparent:-
1) The list of the calendar date [i.e. the month/day part only] of the first eclipse in successive Saros series contains within it sequences of dates which slowly decrease before suddenly increasing again. Though not perfect, this pattern is quite clear and definite and has a periodicity of about 19 series. Fig.3 shows this periodicity for Saros series -13 to 190: the y-axis is the calendar date [corrected for precession and Julian/Gregorian calendar] of the first eclipse of each series expressed as a fraction of a year.
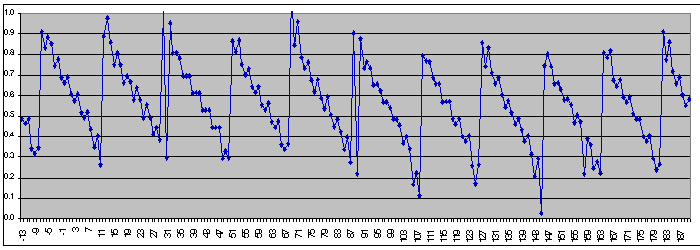 Fig.3
Fig.3
2) The number of eclipses in each phase of a Saros series (opening partials, non-partials, closing partials) is almost entirely determined by the calendar date of the first eclipse. The diagram below shows this dependency: the x-axis runs from January at left to December at the right.
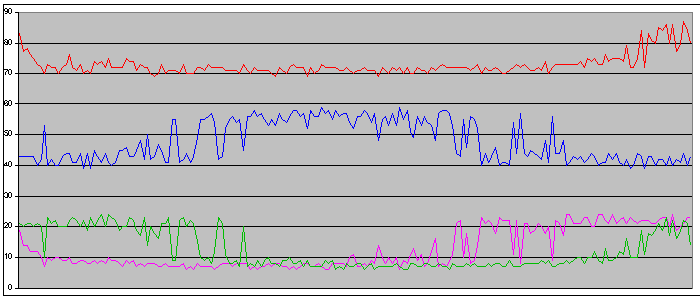 Fig.4
Fig.4
Ignoring the "spikes" [which I shall deal with much later in this article], it is clear that (for example) for a Saros series to have a large number of opening partials, shown in pink, it must start between about August and January. For a series to have a very large number of eclipses overall, shown in red, it must start between October and January, and likewise for other combinations. [When comparing these statements with lists of Saros series, don't forget that these dates are adjusted for precession and calendar change.]
Given the two factors above - that the calendar date of the first eclipse of Saros series listed in numerical order has a regular periodicity, and that the distribution of the numbers of eclipses in the phases of a series is strongly dependent on calendar date - it must therefore follow that the graph of the distribution by Saros series number must consist of repeated sequences of the date-dependency graph (Fig.4). This is exactly what is shown by Fig.5 (an extended version of Fig.1) - click/tap on the figure to overlay a compressed version of Fig.4 for comparison. [Note that because the dates of first eclipse in successive Saros series decrease, to give an accurate comparison I have reversed the x-axis of the overlay - it thus now runs from December on the left to January on the right]. Observe that there are the same number of repetitions of the Fig.4 pattern in Fig.5 as there are cycles in Fig.3 - eleven - and that they occur in the same places.
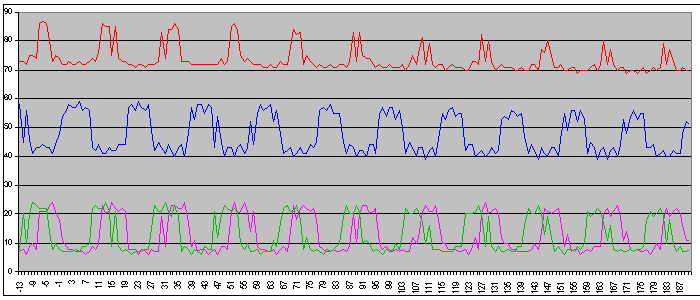 Fig.5
Fig.5
While the above observations give us the reason for the periodicity in the graphs, at the moment this is simply a descriptive explanation based on an analysis of the basic parameters of Saros series. The analysis shows that calendar date is of fundamental importance in determining the distribution of the numbers of eclipses in the various phases of a series but gives no indication as to why. To make progress, we have to embark on a completely different line of enquiry.
To begin the theoretical analysis of Saros series, we can move on to consider the number of eclipses a series "ought" to have. As mentioned above, and further explored in my Theory of Eclipses article, due to the slight differences in the various lunar periods that are each almost exactly equal to the Saros period, a Saros series contains a finite number of eclipses. These begin with partials, become total or annular as the alignments improve and then return to partials as the series ends. The alignments are not just in the numbers of course but also in the physical positions of Sun, Moon and Earth. In the Theory of Eclipses article I introduced the concept of a "window of opportunity", through which the eclipse position moves. When the Moon is barely within the window the edge of its shadow only just touches the Earth - the initial partial eclipses thus occur at very high latitudes. Successive eclipses move nearer to the equator as the alignments improve (i.e. as the Moon moves further into the window), and then away from it again towards the opposite hemisphere. The number of eclipses in an "ideal" Saros series is thus determined by the width of the window within which an eclipse is possible and the rate at which eclipses advance through it.
Tabulated data on eclipses do not, however, normally give a direct measure of the position of the eclipse within the window of opportunity. Instead, the position is defined in terms of the equivalent parameter "gamma", and this is the term I shall use in the rest of this article. The gamma value of an eclipse is a measure of how far the centre-line of the eclipse shadow (in the direction Sun-Moon-Earth) passes north or south of the centre of the Earth, in units of the radius of the Earth. By convention, eclipses passing to the north have positive gamma values and those passing to the south have negative gamma values. A perfectly-aligned eclipse has a gamma value of zero, as the centre-line of its shadow passes directly through the centre of the Earth. It can thus be seen that large values of gamma (whether positive or negative) correspond to eclipses that are just within the window of opportunity and values around zero correspond to eclipses in the middle of the window.
One would think that the gamma value of a total eclipse just grazing the Earth would be (+/-) 1.00 i.e. one Earth-radius from the centre, but due to the fact that the Earth is not a perfect sphere it is actually about 0.9972. Eclipses with gamma values greater than 1 are possible of course - this just means that the centre-line misses the Earth entirely and so nowhere on Earth is the eclipse total i.e. they are "true" partial eclipses (as opposed to being partial because the observer is too far away from the middle of the track on the Earth's surface of a total or annular eclipse). Eclipse gamma values cannot increase indefinitely however, as the error in the alignments eventually prevent an eclipse happening at all (when the Moon moves fully outside the window of opportunity). The largest gamma value for which an eclipse may just happen is about (+/-) 1.57 [the largest actual initial gamma value in Saros series 0 to 190 is 1.5674, for series 108].
A Saros series whose eclipses begin in the northern hemisphere thus starts with partial eclipses having gamma values around 1.57. Gamma slowly decreases with subsequent eclipses until, having crossed the 0.9972 boundary, they become total/annular. Further decreases eventually take gamma across the zero line - the eclipse whose gamma is closest to zero is called the central eclipse of the series - and then into negative territory. As we have seen above this just means that the centre-line now passes to the south of the Earth's centre. The total/partial line is then passed again on the way out before gamma once more gets so large that an eclipse is no longer possible. For Saros series which start in the southern hemisphere gamma begins at around -1.57 and gets larger, ending up at +1.57 at the end of the series. By analysing the geometry of the situation, one can show that the eclipses in Saros series which begin in the northern hemisphere must all be at the ascending node, and those for series which begin in the southern hemisphere must all be at the descending node i.e. odd numbered series begin in the northern hemisphere, even numbered ones in the southern. To see why this should be so, click here. [To avoid the +ve/-ve gamma issue, in the analyses that follow I have reversed the sign of Saros series starting in the southern hemisphere, so the initial gamma value for all series becomes positive. This does not affect the details of the analysis, but makes graphs etc. much clearer and easier to understand.]
The rate at which the Saros series advances is determined by the extent to which the "new Moon" and "node crossing" versions of the Saros are not quite the same number, as this gives the additional mis-alignment of Sun, Moon and Earth after one Saros interval (i.e. one eclipse in the series). This difference is (currently) 0.036days, in which time the Moon moves by 0.478deg relative to the node. To convert this to a difference in gamma requires a bit of spherical geometry involving the inclination of the Moon's orbit (just over 5deg at an eclipse), but suffice it to say that the answer is 0.0422. (Note that although the difference between the "new Moon" and "eclipse seasons" versions of the Saros is much greater, at 0.461 days, the speed at which the node is moving out of alignment [180deg in 173.31days] is much slower than that at which the Moon is travelling relative to the node [360deg in 27.21days]. The overall result is thus the same: a movement of 0.478deg).
The number of eclipses in a Saros series should thus be the width of the window (1.57 x 2) divided by the shift in gamma per eclipse (0.0422), which is 74.41. This is clearly in the correct range (actual numbers range from 69 to 87), and because there are many more Saros series with smaller numbers of eclipses than with larger, the weighted average for actual Saros series is in fact 73.55. Furthermore, this is the average across the several millennia covered by the Saros series involved in the calculation, over which period the synodic and draconic months vary slightly, while the theoretical number uses the values applicable to 2000AD. The theory therefore looks good so far, but we have a long way to go yet!
For there to be any variation in the number derived above it is obviously the shift in gamma (which I shall call delta-gamma from now on) which must change, as the eclipse limits are defined purely by geometry and must therefore be considered fixed. A change in delta-gamma implies a change in the amount the Moon moves relative to the node, which requires it to be moving at a different speed or have a different distance to travel. Given that eclipses a Saros interval apart have almost identical values of Moon-Earth distance (because the synodic and anomalistic months keep in step, as mentioned above), the Moon's speed will be very similar too. The answer must thus lie in the distance the Moon has to travel between the new Moon and node-crossing instants.
The mechanism which can cause this distance to change involves the variable speed of the Earth on its journey round the Sun. Because of the eccentricity of its orbit, it moves slightly faster than average in January, when it is nearest to the Sun [perihelion], and slower in July, when it is furthest away [aphelion]. If the Earth is moving faster, the (imaginary!) line connecting Sun and Earth will rotate through a larger angle in the time the Moon takes to orbit the Earth. New Moon (and hence the eclipse) happens when the Moon crosses this line. The larger angle means that the Moon has further to go to catch up with and cross the line, and so the instant of new Moon will be delayed i.e. the "new Moon" version of the Saros will be slightly extended. This will reduce the difference between the "new Moon" and the (slightly longer) "node crossing" versions of the Saros and so delta-gamma will also reduce. Similarly, when the Earth is moving more slowly the Saros period will be shorter and delta-gamma will increase. Eclipses happening in January will thus have small gamma differences while those in July will have large differences. In fact, with the current amount of eccentricity the extra time the Moon must take to get to the new Moon position in January allows it to almost exactly reach the node again and so delta-gamma is not just smaller but is actually almost zero. Conversely, in July its value is about double the average. The graph of the gamma value of successive eclipses in a Saros series will thus not be a straight line: it will be almost flat when the gamma difference is small and steep when it is large, leading to a markedly "curvy" graph.
Note that if the eccentricity were smaller the aphelion/perihelion effect would also be smaller and so the Moon would not be able to reach the node in time. This would result in the otherwise sustantially flat section of the gamma graph having a small downward slope. Conversely, a larger eccentricity than the current value would generate a larger aphelion/perihelion effect, which would enable the Moon to not only reach the node before an eclipse but to move past it. The gamma difference will thus be not just close to zero for these eclipses but actually negative, and so the graph will slope upwards at this point. This allows the interesting possibility that the value of gamma might move back past an eclipse limit it has just crossed! If this was the eclipse/no-eclipse limit there can be several "non eclipses" until the change in eclipse date decreases the aphelion/perihelion effect sufficiently for delta-gamma to become positive again and thus cause gamma to reduce below the limit once more. The importance of these "second-order" effects will become apparent later on in this article.
We have here the first part of the explanation for the observation that the development of a Saros series is almost entirely determined by the calendar date of its first eclipse. This date determines the dates of all the other eclipses in the series and so if certain eclipses must happen around January or July for the above effect to come into play then this will only be possible for series starting on a very small range of dates.
The variation of delta-gamma can be related back to the "window of opportunity" by remembering that the value of delta-gamma is an expression of the distance between successive eclipses in the window. If delta-gamma reduces, successive eclipses are closer together and if it is larger they are further apart. On a plateau, where delta-gamma is very small, successive eclipses are very close together i.e. they form a "bunch". On a steep section, where delta-gamma is very large, successive eclipses are far apart. Keeping this picture in mind might be helpful in the discussion that follows, as it gives a physical picture of what is actually happening as delta-gamma varies.
One might make the objection at this point that as the Moon's orbit is also eccentric (much more so than for the Earth, in fact) its varying distance from the Earth, and hence its orbital speed, should have a considerable impact on the Saros period. However, don't forget that eclipses separated by one Saros period have very similar values for each of the Moon's orbital parameters, so if the Moon is near to the Earth for one eclipse it will be so for the next also. There will be a slight lunar effect though, as the shift in orbital position relative to perigee from eclipse to eclipse in a series (though small) is not zero. In fact it is (currently) 2.82 degrees - compare this to the 10.67 degrees relative to perihelion that the Earth will move in the same interval. In an "average" Saros series of 75 eclipses the cumulative shift will thus be just over 211deg for the Moon compared to 800deg for the Earth. Any apogee/perigee effect will thus complete a little over half a cycle during a complete series while the aphelion/perihelion effect will complete almost 21/4 cycles. The variation in the Moon's speed will therefore add a long-period fluctuation to the graph rather than the plateaux and steep sections produced by the variation of the speed of the Earth.
By comparing Saros series that have almost identical start dates but different lunar apogee/perigee histories, it is clear that gamma differences reach a somewhat larger maximum value when the Moon is near apogee and a smaller maximum value when it is near perigee. This means that steep sections of the gamma-value graph spanning apogee will be steeper than when they span perigee. A steeper section will increase the distance between the plateaux before and after it, possibly moving the one after it across either the upper or lower total/partial eclipse limit or the lower partial/none limit. The first case will result in the series having different numbers of non-partial eclipses compared to opening or closing partials than would otherwise have been the case, the second will result in there being fewer closing partials. A similar effect is seen for the minimum gamma difference value but this has less effect on eclipse numbers, as a small tilt on an already flat plateaux will not alter things so much. I shall explore this phenomenon in (much!) greater detail later on in this article.
Every eclipse in a given Saros series is, of course, one Saros period later than the one before. As the Saros period is 18yrs plus 10 or 11 days (depending on how many leap years are spanned) and 8hrs, the date in the year of successive eclipses advances through the calendar by 10 or 11 days each time. Although this is a relatively slow process, there are (as shown two paragraphs back) sufficient eclipses in a series for their calendar dates to move through an entire year more than twice over. This means that the aphelion/perihelion effect described above will run through more than two cycles of large and small gamma differences, leading to there being two "curves" in the graph not just one - the diagram below illustrates this phenomenon.
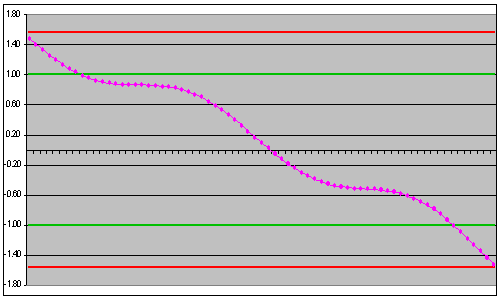 Fig.6
Fig.6
Fig.6 shows the value of gamma at the successive eclipses of Saros series 97. The red lines indicate the limits beyond which an eclipse is not possible (+/-1.57), the green ones the boundary between partial and total eclipses (+/-0.9972). Note the two sections of almost constant gamma and the alternating sections of rapidly-changing gamma. In "window" terms this will produce two bunches, each centred on the section of constant gamma.
Given that a Saros series will end when the gamma value moves outside the +/- 1.57 limits, a flat section will increase the number of eclipses in a series (as here the gamma value makes no progress towards a limit) and a steep section will decrease the number (as here the gamma value moves quickly towards a limit). This process could change the number of eclipses in a Saros series from the "ought to have" value calculated above, but of course if every series always had the same number of flat and steep sections the total number of eclipses would still be the same from series to series. In practice, this is not the case but before exploring this aspect further I need to explain a process which is central to the whole problem.
As can be seen from the shape of the Panorama sections given in Fig.2 and Fig.2a above, between the sudden jumps the top edge descends at about 45degrees i.e. the start date of successive "regular" Saros series is offset by, on average, about one Inex period plus one Saros period. In fact, taking data from actual Saros series, the true average interval between regular series in the part of the Panorama shown is about 44.2yrs. Now an Inex is 28.94yrs and a Saros is 18.03yrs, so 44.2yrs is pretty close to one Inex plus one Saros - given that the "1 Inex" bit is fixed (by the definition of the Saros-Inex Panorama), it is actually 1 Inex plus 0.844 of a Saros. Now of course you can't have "0.844 of a Saros", so this value must be interpreted as an average over a number of intervals e.g. if in a series of 13 intervals 11 of them were "I+S" but 2 were just "I", the total interval would be 13I+11S and so the mean would be I+0.846S. On this interpretation, because an Inex is 20.3 days less than a whole number of years and a Saros is 10.8 days longer (taking average values), the mean value of 1 Inex plus 0.844 of a Saros is 11.2 days less than a whole number of years. The start date of successive regular Saros series will therefore, on average, move backwards through the calendar by just over 11 days per series. If the start date is earlier, each of the eclipses in the series will also be earlier and so each will experience a slightly different amount of aphelion/perihelion effect as compared to the corresponding eclipse in the previous series. This will result in a slightly altered value of delta-gamma for every eclipse in the series and so the graph of successive gamma values will also be different. It will not be different in overall shape though, as the cycles of large and small delta-gamma will be traversed in the same way irrespective of the actual start date. What does change is the graph's "position on the page", as can be seen from Fig.7.
 Fig.7
Fig.7
Here we have the gamma-value graphs for Saros series 37 (purple), 40 (dark blue), 43 (light blue), 46 (green) & 49 (orange), plotted in the correct chronological order of their initial eclipses. I hope it is clear that each successive graph has the same basic shape but moves "right and down" relative to the one before.
I have derived some mathematical descriptions of this process, which can be found by clicking here. It is not necessary to understand the maths to appreciate later explanations however, so I shall return to the graph for series 97 because we are on the brink of an important explanation.
Fig.8 shows the graph for series 97 again, but adds the graph for series 94 (in blue). It should now come as no surprise to see that the two graphs are offset, with that for series 94 being above and to the left of that for series 97. More subtly, however, whereas the first flat section for series 97 lies below the partial/total line, the shift between them means that the plateau for series 94 lies above it. Series 94 should thus have many more opening partial eclipses in its sequence than series 97, and this is born out by actual data - series 94 has 21 while 97 has only 8.
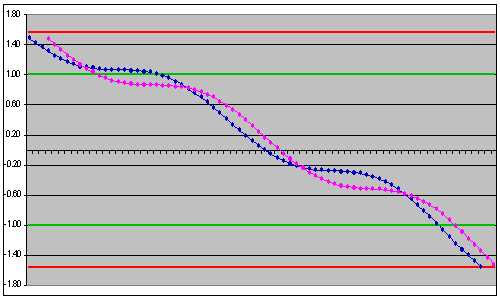 Fig.8
Fig.8
We thus have here a mechanism whereby the number of opening partial eclipses can change. It will also apply to closing partials of course, as the same movement across the partial/total limit can occur at the other end of the graph. What should be clear, however, is that because the distance between the two plateaux is much less than the distance between the two partial/total limits, this mechanism can never affect both the opening and closing sequences at the same time. Either the first plateau will be crossing the upper limit or the second will be crossing the lower limit but both cannot be crossing simultaneously. This accounts for the fact that, while the graphs of the number of opening and closing partials in Fig.1 have the same shape, they are shifted relative to each other i.e. only one graph changes at a time. Also, because the mechanism does not change the basic shape of the gamma-value graph, just where the plateaux lie relative to the limits, the total number of eclipses cannot be changed by it. This means that if, as in this case, a series suddenly loses many opening partials then its number of non-partials will be much larger to compensate. This can be seen by examining Fig.8 and is also born out by actual data - series 94 has 44 non-partials while series 97 has 57, the difference being exactly that between their numbers of opening partials. The mechanism by which this compensation takes place is pretty obvious - any given eclipse can only be either above the limit or below it and so any loss of opening partials (above the limit) will add to the non-partials (below the limit). This explains why the non-partials total is only large when both partial-phase totals are small - this requires both plateaux to be within the partial/total limits.
What might not be quite so obvious is that the transition from a large number of opening partials to a much smaller number will be very abrupt. This is due to the flatness of the plateaux, this itself being caused by the many almost-zero values of delta-gamma in this area. It will thus take only a small shift of the graph for many points formerly above the partial/total limit to suddenly be below it. In fact, the passage of just one series is sufficient because the shift between successive series is much more than the "non-flatness" of the plateau (I showed this on the maths page concerning shifts between series, if you read that bit). This is the reason that the number of opening and closing partials basically takes just two values and switches between them almost instantly: one shift is sufficent to bring all the eclipses constituting an entire plateau over a limit. The fact that the "large" and "small" values are the same for the two phases is down to the symmetry of the situation. Both plateaux are caused by the same aphelion/perihelion effect and although there will be small changes in the magnitude of this process during the over 600yrs between the eclipses constituting each plateau these will not be significant compared to the shift between series.
We have already seen that the number of eclipses in each phase of a Saros series is almost entirely determined by the calendar date of the first eclipse - Fig.8 gives a further insight into this dependency. For the opening or closing partials phase to be long the series must not only have an initial or final plateau but the plateau must happen when the gamma-value graph is still outside the partial/non-partial limit line. The calendar date of the eclipse at the centre of the plateau must be (approximately) at perihelion [i.e. January] and so the date of the first eclipse can only fall within quite a small range if the plateau is to be in the right place. Similarly, for the non-partial phase to be long the middle eclipse of the series must be close to aphelion [i.e. July] to ensure there is a steep section near the centre of the graph, thus allowing both plateaux to fit within the limits. Clearly, therefore, there will only be a certain range of dates when a Saros series can start such that its various phases can span perihelion or aphelion and thus give them the appropriate numbers of eclipses - this is what we see in Fig.4.
I said above that the shift in the graphs for successive Saros series does not affect their basic shape, but this is only true up to a point. It is important to realise that the gamma-value graph does not actually stop when gamma goes outside the eclipse limits. The various orbital periods will still have the same interactions as they did before, but once the offsets between them have become quite large there will be no actual eclipses. Gamma will continue to increase, but it will also undergo the same variations due to the Earth's changing orbit speed as it did when there were eclipses, and so there will still be flat and steep regions. As the various periodicities get further and further out of step, gamma will keep increasing until the alignment of Sun, new Moon and Earth is "maximally bad", at the point when the Moon is as far from a node as it can be. After this, gamma will decrease as the alignments steadily improve. Eventually, eclipses will begin again and a new series will start, but this time at the other node from previously as the position of the eclipses will have moved round an entire half-orbit.
One could of course argue that the "new" series is not truly new but simply a re-vitalisation of the old series after a long period when it was dormant. Given that the sector at each node within which an eclipse is possible is about +/-18deg (see my article on Ecliptic Limits!), on this basis an "eternal" Saros series is dormant for fully 80% of its life: the interval between active phases is thus a little over 5,000yrs. Prompted by a section contributed by Kurt Leingaertner in the fifth volume of the 'Mathematical Astronomy' books by Jean Meeus, I explored this idea of Saros series being eternal and came up with some surprising results, which I've placed on a "maths" page - click here to be surprised!
In terms of gamma graphs, the significance of the curve existing outside the eclipse limits is that if it is shifted up or down enough (due to a change in the series start date), a flat section that was formerly "out of bounds" can be moved within the limits and thus affect the total number of eclipses in the series. This is vital to the whole discussion!
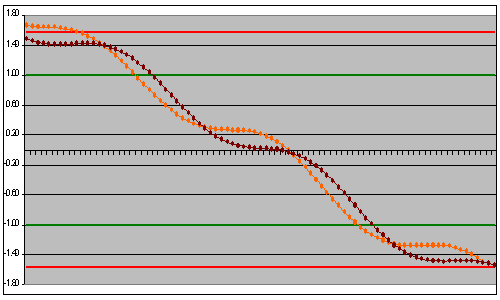 Fig.9
Fig.9
Fig.9 shows the curve for series 49 again (in orange) but this time extended "backwards in time" to include points with gamma greater than the eclipse limit (1.57). As can be seen, this has revealed a plateau sitting just above the limit. The curve for series 52 is shown in brown. It is (as with other examples) shifted down and right relative to that for series 49, so the plateau that was "out of bounds" for series 49 has now come into play for series 52 as all its eclipses now have gamma values lower than the limit. Not only that, but the lowest plateau is still within the -1.57 limit and so we have a curve with three plateaux (and thus three bunches) but only two steep sections. The plateaux thus add more extra eclipses than the steep sections subtract and so the overall total number of eclipses should have increased. This is, once more, supported by the data: series 49 has 72 eclipses while series 52 has 86. Note also that the plateaux are in such a position that series 52 has large numbers of both opening and closing partials. Due to the partial/non-partial "mutual compensation" effect discussed above this is, in fact, the only way that any series can have a large number of eclipses overall.
It should be clear that it wouldn't take much more of a shift before the lowest plateau of series 52 moved below the -1.57 limit, removing the eclipses on this portion of the curve from the total and thus reducing it to the same number as there were before the "new" plateau at the top came into the reckoning. This explains why there are only a few series with the larger number of eclipses - the "leeway" between an extra plateau appearing and a previous one departing is relatively small. We can do some simple calculations here to work out the numbers, which I have once more placed on a "maths" page: click here to read all about it.
Note the similarity between Fig.9 and Fig.8 by the way, emphasising that it's not a change in graph shape that's important but rather its position relative to the limits.
Taking all the mechanisms described above together, we can now construct a full explanation of the variabilities in both the partial and non-partial phases of a Saros series.
I firstly showed that, because the slight mis-alignments in timing between the various lunar cycles shift the position of the eclipse centre-line relative to the Earth for each eclipse in a series, the "history" of a Saros series can be represented by a graph of the gamma value of each eclipse. I then described how the variable speed of the Earth on its orbit round the Sun resulted in a regular fluctuation in the difference in gamma value between successive eclipses, and how this caused the shape of the gamma-value graph to have "flat" and "steep" sections. Finally, I showed how the presence of these sections could influence the number of eclipses in each phase of a series.
The key to the variability was found to be the "down and to the right" shift of the graphs of successive series due to the earlier and earlier calendar dates of their initial eclipses causing them to experience different amounts of gamma-value variation. These shifts cause the flat sections of the graph to move across the limits for partial and total/annular eclipses and thus change the numbers of such eclipses within the series.
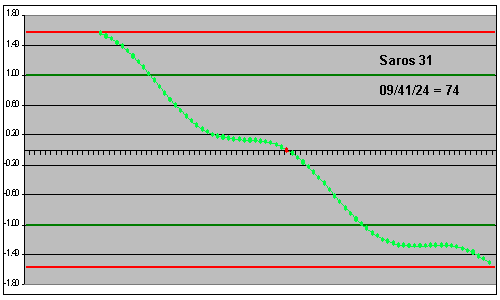 Fig.10
Fig.10
Fig.10 illustrates how the variabilities come about. It shows the gamma-value curve for every third Saros series from 31 to 55 - keep clicking/tapping on the diagram to view all the graphs in the sequence. The distribution of opening partials, non-partials and closing partials is shown on each graph, together with the total number of eclipses in the series.
The first series in the sequence, number 31 is a "standard" series, with a small number of opening partials, a large number of closing partials and hence the smaller number of eclipses overall. The next series, 34, shows the "3 plateaux plus 2 steep" structure discussed in the immediately previous section so has large numbers of both opening and closing partials and thus a large number of eclipses overall. Series 37 still has the larger number of opening eclipses but the third plateau has disappeared as it has been shifted beyond the lower eclipse limit. This series therefore has the smaller number of closing partials and thus a lower number of eclipses in total. Series 40 is interesting, as its upper plateau lies almost exactly on the partial/total limit and so some of these eclipses remain as partials although the majority are non-partial (annular, in fact). This accounts for its slightly unusual number of opening partials. Series 43 is fully into the "small number of opening and closing partials" regime, while the lower plateau for series 46 has moved below the lower limit and so it has a larger number of closing partials - its overall total is still low, however, as the switch to a larger number of partials is compensated by a resultant lower number of non-partials. Series 49 we have seen before, with its hint of an approaching plateau at the top, and finally series 52 regains the 3 plateau structure and so, as we would expect, has a larger number of eclipses overall. Series 55 is then almost a repeat of series 37, losing the bottom plateau and hence having the lower number of eclipses in total.
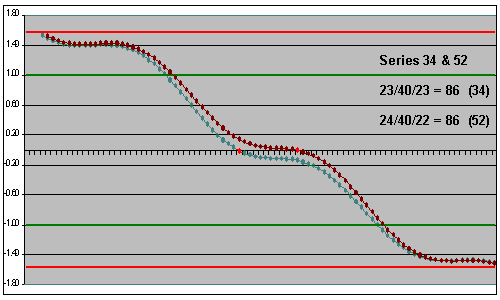 Fig.10a
Fig.10a
It is instructive to compare series 34 and 52, as shown by Fig.10a, above. Their top and bottom plateaux are in exactly the same position and the middle sections are offset by only a small amount considering the large numerical difference between the series numbers. This observation is illustrative of the fact that there is a periodicity of about 19 cycles in the characteristics of Saros series, which can be seen in Fig.1 and also in Figs.2 and 2a. I shall have more to say about this when I derive some numbers later on. [I shall also explain why the slight offset exists - it's actually to do with the varying distance of the Moon from the Earth]
To show that the gamma-value curves used above do indeed generate graphs with the shape given in Fig.1, I have plotted the numbers of partial and non-partial eclipses for the series illustrated in Fig.10 - the colours used are the same as those in Fig.1.
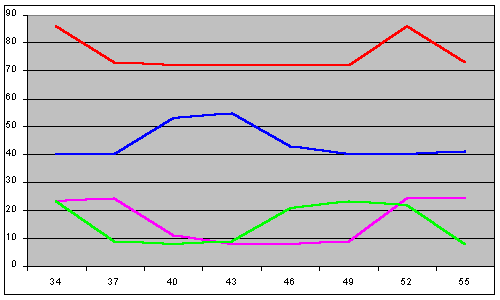 Fig.11
Fig.11
Pretty conclusive, I think! Rather more "chunky" than Fig.1 of course, due to the use of only every third series, but clearly the right shapes. I feel I can thus now claim to have explained (in a descriptive sense, anyway) the "variations in the composition of successive Saros series" as mentioned by Meeus. This is by no means the end of the story though - far from it!
Something which comes out of a close study of Fig.10 is that the position of the first eclipse of series 34 to 49 steadily moves from left to right but then jumps back to the far left for series 52 before moving right again for series 55. Given that the horizontal axis is [effectively] a measure of time, as each eclipse in a series is later than the one before, this means that the start date of successive series gets later and later (as one might expect) before, rather counter-intuitively, suddenly jumping to a much earlier date. [This "flip-back" applies to both the full date and the calendar date, by the way]. The reason for this is straightforward: if a series is to have another 12 or 13 eclipses at its start yet keep the date of the eclipse at the top of the steep section only slightly later than the corresponding one in the previous series, then clearly these extra eclipses must happen at much earlier dates. An alternative view is that, given the observation made a few sections above that the gamma-value curve still exists above the "no eclipse possible" limit, if fuller curves were plotted for each series it would be clear that concentrating on the point at which the curve crosses the upper limit is somewhat artificial. By considering the wider picture it would be obvious that all the curves simply move down and right in sequence (rather like an extended version of Fig.7, in fact).
This can be seen more clearly where the curves cross the partial/non-partial limit. The same "flip-back" in fact happens to the dates of eclipses here, but because this segment is within the body of the series it doesn't attract attention. More importantly, the effect is also seen at the point at which the curve cuts the gamma=0 line, which rather upsets the commonly-held view that Saros series are numbered according to the date of their "most central" eclipse (i.e. the one with gamma nearest to zero). This will be true for series with the lower number of eclipses, as the point at which their graph cuts the gamma=0 line will steadily move from left to right, but around the time when the higher number of eclipses appears this steady advance is interrupted. Run through the graphs in Fig.10 again - I have marked each most-central eclipse in red. It is clear that while the red dot moves forwards each time from series 34 to 49 it jumps considerably backwards between series 31 and 34, and then again between 52 and 55, as the middle plateau crosses the gamma=0 line.
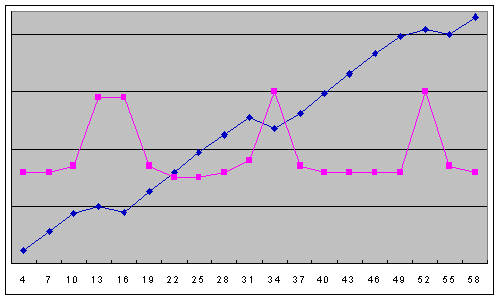 Fig.12
Fig.12
Fig.12 is a plot of the date of the most-central eclipse [blue] and total number of eclipses [pink] for a sequence of series of which those used as examples above are part. It is clear that for "ordinary" Saros series the date of the most-central eclipse increases nicely with series number but when a series with a large total number of eclipses turns up it causes a dip in the graph due to the date of the most-central eclipse moving backwards in time, as demonstrated.
In my explorations so far I have been careful to restrict myself to the consideration of Saros series active in centuries well before the current epoch. This is because the variabilities in the composition of series are themselves variable! This was pointed out by Meeus in the fourth volume of his Mathematical Astronomy Morsels series, when he presented a version of the diagram I give as Fig.13.
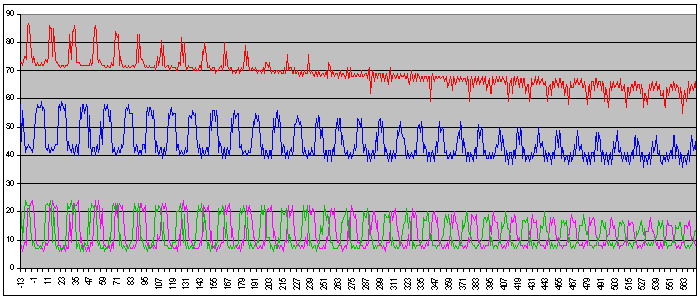 Fig.13
Fig.13
This is Fig.1 extended slightly back in time (to about 3300BC) but a long way into the future - the right-most series (number 575) will not begin until around 13500AD!! As a guide to timescales, the series active at the current time run from 117 to 155.
It is clear that once one goes beyond series 200 or so, strange things begin to happen. The number of series with large totals of eclipses falls dramatically, then disappears altogether, and then the peaks turn into troughs! The reason for this can be seen in the lower two graphs, of opening and closing partials.
In my discussion of the "down and right" shift of gamma-value graphs I pointed out that the only way a series can have large numbers of eclipses overall is to have large numbers of both opening and closing partials. Inspection of the green and pink graphs shows that from about Saros 200 onwards these graphs no longer overlap i.e. after this point at no time do large numbers of opening and closing partials occur together. There can thus be no series containing large numbers of eclipses. To the far right, there is even a gap between the two graphs i.e. we now have regions where low numbers of opening and closing partials occur together. This will produce series with unusually low numbers of eclipses overall, as observed in the top graph. To understand why the partials graphs slowly move apart as time passes we must consider how the orbit of the Moon changes.
At the beginning of this article I explained how the slight discrepancy between the "new-Moon" and "node-crossing" versions of the Saros period causes successive eclipses in a series to have an average gamma-value difference of, currently, 0.042. This average difference is, however, not constant over time because the various orbital periods of the Moon are very slowly changing. There are three main causes: perturbations from the Sun and the other planets (including the fact that the Earth is not a perfect sphere); the consequences of the oceanic tides; and the changing eccentricity of the Earth's orbit round the Sun.
Changes in the lunar orbit due to the influence of the Sun are pronounced but tend to be periodic and of very short period (from tens of days to small numbers of years). They thus only have a minor effect on the variations under consideration here. All bodies in the solar system suffer perturbations from the other bodies but this is only significant for objects such as Moons and asteroids, due to their relatively small size. The Earth-Moon system is particularly affected by Venus, as it is both close and large (for a non gas-giant planet, anyway). As well as being periodic (but of relatively short period) the effects are also "secular" i.e. tending to act in a constant direction over a long period of time, and so can have an effect over the timescales we are considering.
The tides have an effect because the frictional force between the land and sea as the Earth's surface rotates through the "tidal bulge" caused by the Moon drags the bulge forward a little (i.e. in the direction of the Earth's spin). This means that the gravitational pull that the bulge exerts back on the Moon is directed very slightly in the direction of the Moon's orbital rotation. This increases the Moon's orbit speed which causes it to slowly spiral away from the Earth, thus increasing its orbital period. Note that at the same time as the Moon is speeding up, the energy lost in tidal friction causes the Earth's rotation rate to slow down, leading to a very slow increase in the length of the day. The "days" mentioned in this section are thus expressed in terms of the current day length, not what it will be at those times.
I will consider the consequences of the changing eccentricity of the Earth's orbit in a separate sub-section, as this produces slightly different effects to the other types of perturbation.
Although the planetary and tidal effects are all small in absolute terms, they are not negligible over a long time-period. The "new-Moon" period was 29.530578 days around 2000BC, is 29.530589 days now and will be 29.530594 days around 4000AD. Not a great difference, to be sure, but when it gets multiplied by 223 to give the Saros period it starts to add up: these values are 6585.3189, 6585.3202 and 6585.3224 days respectively which means the "new-Moon" Saros period increases by 5 minutes in 6000yrs. The "node-crossing" Saros period also gets longer, but by a slightly larger amount each time which results in the difference between the two Saroses, and thus delta-gamma, increasing. Because the values of the two Saroses are almost the same, a small change in their absolute values results in, proportionally, a much larger change in the difference. Mean delta-gamma thus in fact increases from 0.040 in 2000BC to 0.043 in 4000AD. Again, not much numerically but this is actually a 7% change - quite enough to noticeably affect the gamma-value graphs.
The effect of an increase in mean delta-gamma is to increase the distance between the plateaux on the gamma-value graph (as there is a greater distance between the points making up the steep section between them). This will reduce the number of series with large totals of eclipses, as it will take fewer shifts to move the bottom plateau over the lower eclipse limit after the "new" plateau has appeared at the top of the graph. There will then eventually come a critical point when the distance between these plateaux is exactly the same as that between the upper and lower eclipse limits. At this point the bottom plateau will disappear just as the new one appears, and so there will be no opportunity for a series to have large numbers of both opening and closing partials i.e no more "long" series. Each shift will bring in the same number of "new" eclipses at the upper eclipse limit as there are existing eclipses leaving the sequence by crossing the lower limit. All series will thus have either a large number of opening partials or a large number of closing partials (but not both), and so the overall number of eclipses in a series will stay more-or-less constant, as seen in the central section of Fig.13. Note, however, that this constant value will itself have been slowly decreasing (if that's not a contradiction in terms!) as the increase in delta-gamma means that fewer and fewer eclipse-to-eclipse intervals can fit within the [fixed] eclipse limits. This is shown by the gradual decrease in the "baseline" levels of overall eclipse numbers.
To calculate when the "constant number" regime might begin, one could consider the situation when the distance between plateaux (which can be calculated) becomes equal to the eclipse limit: when this happens, it will no longer be possible for there to be three plateaux within the limits. However, because the "effective value" of the eclipse limit is somewhat arbitrary (as the gamma value of the first eclipse of a series varies quite widely), it is not possible to make firm predictions this way. It is better to consider the number of eclipse intervals in a plateau-to-plateau cycle: this value is accurately calculable and virtually constant (as it only depends on the length of the Saros period which, as we saw above, only changes very slightly over time). As calculated in an earlier section, this value is 34.30. When three plateaux only just fit within the eclipse limits, there must therefore be (2 x 34.30) +1 eclipses involved [the "plus 1" arises because 34.3 is the number of intervals between eclipses, not the number of actual eclipses]. This comes to 69.6, which Fig.13 shows us is indeed the number of eclipses per series at which there begin to be very few series with the larger total number of eclipses.
As delta-gamma, and thus the distance between plateaux, gets increasingly large there will come a point when a shift will move the bottom plateau fully over the lower limit before the top one has come within the upper limit i.e. neither plateau will be within the limits and so their eclipses will not contribute to the series totals. This will result in a series with low numbers of both opening and closing partials and hence a very low number of eclipses overall. These series will initially be in the minority, as the upper plateau will soon come into play, but as delta-gamma grows ever larger the time it takes to do so will increase and so the number of very-low-total series will get bigger, all as seen in the far right-hand section of Fig.13.
The reason for the aphelion/perihelion effect being present at all is the changing speed of the Earth on its orbit, due to its varying distance from the Sun. This variation is caused by the eccentricity of the Earth's orbit, so if this changes so will the magnitude of the aphelion/perihelion effect. In fact, the eccentricity is currently decreasing - in 2000BC it was 0.01818, it is now 0.01671 and in another 4000yrs it will be 0.01483. By the time we get to the right-hand side of Fig.13 it will be only 0.01062. This will cause the aphelion/perihelion effect to also decrease over this period, which will reduce the variation of delta-gamma from the mean i.e. the large values will be smaller than they would otherwise have been and the small ones will be larger. The effect on our gamma-value graphs will be to make the steep sections less steep and the plateaux less horizontal - the whole graph will thus become less "curvy". This will mean that the transitions between the larger and smaller numbers of eclipses in a phase will become less sudden (as the non-horizontal plateau regions will now gradually slide over the limits rather than quickly jump) which will then affect the transitions between the "no large totals", "all series the same", "some low totals" and "mainly low totals" regimes described above. Eventually, as the eccentricity gets very low, the graph will be almost a straight line and so, because there are no longer any steep or plateau sections, all series will have the same number of eclipses (quite a small number of course, due to the much larger value of delta-gamma at this time).
Apart from the effects described above, the changing eccentricity also has other, much smaller, second-order effects based on the same mechanism as that which smooths out the gamma curves of high-numbered series: the eccentricity affects the slope of the plateaux. We have seen above that when the eccentricity is small the plateaux are less pronounced, and of course the converse is also true: when the eccentricity is large delta-gamma can be negative, resulting in upwards-sloping plateaux. The number of eclipses in a series is determined by how quickly gamma proceeds from one eclipse limit to another. Downwards sloping plateaux will speed the progression, upwards slopes will (temporarily) reverse it and so, other things being equal, a series running at a time when the eccentricity is large will have more eclipses than one running when the eccentricity is small. In practice however, the difference will be slight. For a non-eclipse to be included in the total its gamma value would have to be reduced by a reasonable amount, as it will generally be sitting on a steep section of the graph where delta-gamma is comparatively large. It is thus unlikely that more than one eclipse will be "dragged back over the limit". Let us also not forget that while a large eccentricity will cause the plateaux to slope upwards due to an increased effect at perihelion, the correspondingly increased effect at aphelion will tend to stretch out the steep sections between the plateaux. This could lead to an eclipse being pushed over the limit and so turn it into a "non-eclipse". The two effects thus tend to cancel out unless there are more plateaux than steep sections (or vice-versa).
A further complication can arise if an "upward-tilted" plateau is sitting close to the eclipse limit. The tilt can be sufficiently great that the end of plateau can be pushed back over the limit, causing a number of non-eclipses until the gamma-curve moves downwards again. The result is a gap in the series, and of course a reduced number of eclipses overall. This effect can be seen in Saros series -88, -166, -188, -227, -243 and -282, resulting in the loss of as many as 9 eclipses. The reduction is specific to these particular series, however, not a general phenomenon.
Many of the behaviours described above are demonstrated graphically by the animations below. These were produced by a model I constructed to investigate the effect of varying delta-gamma. The basic curve is taken from an actual Saros series but the intervals between the data points can be systematically altered to simulate the effect of changes to both delta-gamma (caused by variations in the lunar cycles) and the aphelion/perihelion effect (due to changes in the eccentricity of the Earth's orbit). The resultant graph can then be shifted up and down to simulate successive Saros series, and the number of eclipses in each phase of the series counted and displayed to top right [note that the left-to-right shift seen in actual series has been suppressed for convenience]. Click/tap on the graph to run an animation.
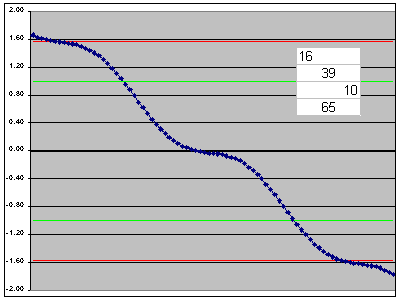 Fig.14a
Fig.14a 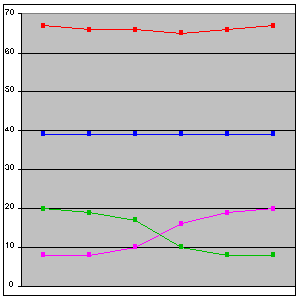
The first animation shows the "constant total" regime, where the upper and lower plateaux barely fit between the eclipse limit lines due to the increase in delta-gamma. Each plateau is also less horizontal than previously, because of the decrease in eccentricity of the Earth's orbit. Note that as eclipses in the bottom plateau move over the lower eclipse-limit line (in red), just the same number appear over the top limit line from the upper plateau. The overall total is thus almost constant. This is shown on the accompanying plot of the number of eclipses in each phase (red=overall total, blue=non-partial, pink=opening partials, green=closing partials). Note that the opening and closing partials lines cross at the half-way point of their rise and fall, thus ensuring that their sum (and thus the overall total) is constant.
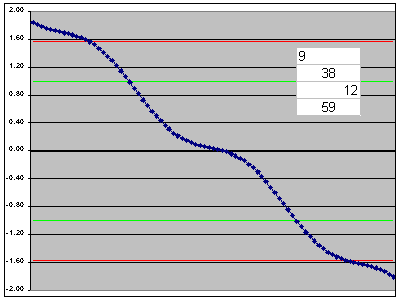 Fig.14b
Fig.14b 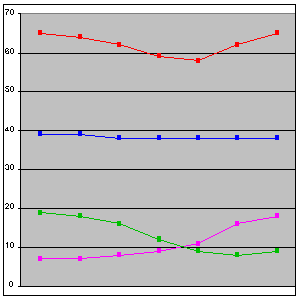
The second animation shows the "diminished total" regime, where the upper and lower plateaux cannot both fit between the eclipse limit lines: the plateaux are also even less flat than before. This time, there is a period when eclipses that move over the lower eclipse-limit line are not compensated for at an equal rate by "new" ones appearing over the top limit. The overall total thus reduces during this period. Again, this is shown on the accompanying plot of the number of eclipses in each phase. Note that the opening and closing partials lines now cross well below the half-way point of their rise and fall, thus producing a period when their sum, and so the overall total, decreases.
I also investigated the situation in the far future, when the eccentricity of the Earth's orbit becomes much smaller than it is now - the minimum value, 0.0023, will be reached in about 30,000AD. Although it is not really sensible to extrapolate so far into the future, I did set up my model with an estimated value of delta-gamma for this epoch to see what would happen. The result was exactly as predicted earlier - the gamma curve became almost a straight line so there was virtually no difference between the number of eclipses in any Saros series. The distribution of partials and non-partials was a constant 10/35/10, giving an overall total of 55.
Finally, I set things up for around 10,000BC, when delta-gamma was much smaller but the eccentricity was much larger. This was to check the earlier conclusion that the larger value of eccentricity at that time could cause a small modulation to the overall number of eclipses. The result was that with the eccentricity unchanged the number of eclipses was 90 and with increased eccentricity it was 91. I haven't done an animation of this, however, as it's not very dramatic!
The difference in delta-gamma variation between the constant total and diminished total regimes is, by experiment, quite small but even so it corresponds to about 150 Saros series. However, there is only a comparatively small section of the overall totals line in Fig.13 which is "almost flat" and so it is not clear that there really is a "constant total" regime at all. The reason for this is connected with the influence of the eccentricity of the Moon's orbit, in a similar way that the eccentricity of the Earth's orbit produces the aphelion/perihelion effect already described. To fully explain this, we finally have to get round to discussing the full implications of the fact that the new Moon and apogee/perigee cycles will only be in step once every three Inexes, mentioned when I introduced the Inex near the beginning of this article. I shall tackle this topic after I have explored some of the less obvious ways that the number of eclipses per Saros series can be affected.
In 2006, Luca Quaglia, originally from Italy but now living in New Zealand, used a program called Solex (written by Prof. Aldo Vitagliano of the University of Naples) to calculate the complete list of solar eclipses taking place from 18,000BC to 22,000AD. In terms of Van den Bergh's Saros series numbers, this is from series -400 to series +720. Solex is a well-known program for calculating the position of heavenly bodies using a process called numerical integration. This takes a given starting position and uses the physical laws of gravitation to work out where everything will be a little later - a very slow and computationally intense process but one which gives very accurate results. I do not have access to this data, but Jean Meeus used it to plot the number of eclipses in each series. I show it as Fig.15 - an extension of my Fig.13 (mainly in the BC direction) but showing only the total number of eclipses per series (i.e. the red line in Fig.13). The time-scale runs from about 16,000BC to 19,000AD i.e. the present epoch sits in the middle of the graph.
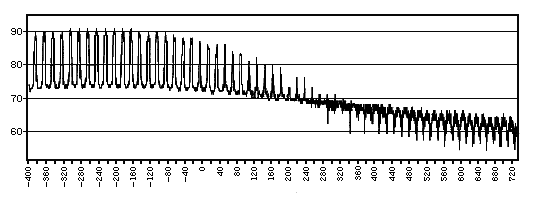 Fig.15
Fig.15
The graph shows a gradual downwards curvature of the baseline number of eclipses as we move into the future (as previously predicted), but there is an unexpected "hump" in the distant past at around series number -230. Data calculated by Kurt Leingaertner [a contributor to the "Morsels" series] indicates that the average mid-year of the two series with large numbers of eclipses centred around -230 (numbers -224 and -246) is 9938BC [Note, by the way, the difference of 22 in the numbers of these series, justifying the comment made elsewhere in this article that the periodicity of series with large numbers of eclipses was longer in the distant past than it is now]. The processes described above could not explain the hump, and so I undertook further research in the hope of determining why it might be present.
My first idea was that it might be related to a change in the recession rate of the Moon due to a reduction in tidal activity. The current rate of increase in the size of the Moon's orbit is about 3.8cm/yr, but if the Moon had been receding at this rate since its formation it would now be much further away: the rate must therefore have been lower in the distant past. There are two possible reasons for this. Firstly, if the continents were distributed differently on the Earth's surface this would affect the tidal friction and if there were less water all aspects of the tides would be altered. The first cause would only apply in the very distant past (due to the movement of the Earth's tectonic plates) but the second could be caused by such things as Ice Ages, which not only lock up a lot of the Earth's free water but also change the profile of the continents due to the lower water levels. A reduction in free water would decrease the tidal bulge and the extent of the tides, and a change of continental profile might well reduce the tidal friction. Both could have thus reduced the Moon's recession rate. The last Ice Age was around 10,000yrs ago, about the same time as the maximum of the hump, so a connection seemed possible.
I was able, through the good offices of Jean Meeus, to contact Luca Quaglia and put my ideas to him. He was interested, and very generously offered to re-run his Solex calculations but with the tidal effect removed. The result is shown below:-
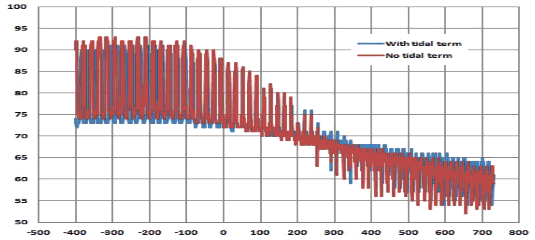
©Luca Quaglia 2011
It can be seen that, far from causing the number of eclipses in a series to fall as we go into the past, the removal of the tidal component actually causes the number to rise slightly! It is also noticeable that the sharp peaks in the two graphs steadily get out-of-step with each other as we move back (or forward) in time. This is due to the timing of eclipses being slightly altered once the change in lunar orbit period caused by tidal recession is removed. The overall number of eclipses will be unaffected, but the timing offsets cause them to be distributed amongst the series differently, leading to different series having large and small numbers of eclipses.
Intuitively, one might think that the recession of the Moon would cause delta-gamma to increase with time. A lower rate of recession would mean a slower rate of increase, which would have needed larger values of delta-gamma in the distant past in order to get to the present value. This would result in a smaller than predicted number of eclipses per series at early epochs, as required. However, this is not actually the case. Because of the different effects it has on the synodic and draconic periods, the recession of the Moon actually tends to decrease delta-gamma. It thus simply slows the rate at which delta-gamma naturally increases due to other causes such as the perturbing effects of the planets. A reduction in recession rate in the past would therefore require delta-gamma to have been smaller then, not larger, and so would increase the average number of eclipses in Saros series at very early epochs rather than decrease it - as shown by the graph. We can thus be certain that reductions in the recession rate due to Ice Ages were not the cause for the decrease in numbers to the left of Fig.15 which produce the "hump".
 The earlier analysis of the effect of changes in the eccentricity of the Earth's orbit was somewhat "descriptive" in style and, in particular, assumed that the eccentricity will continually decrease with time. However, although the eccentricity is indeed currently decreasing, this has been so only since about 10,000BC: before that it was increasing. After about 30,000AD it will again begin to increase and so over the timescale of my Saros Series investigations the eccentricity displays "pseudo-periodic" behaviour (see figure to the right). Given that the maximum in this graph is at the same period in time as the hump in Fig.15 it is tempting to ask what effect the variation in eccentricity might have on the numbers of eclipses in series at these distant epochs. To investigate this we shall have to move from a descriptive approach to one based on calculation - this is where the "indirect" effects of the eccentricity changes make themselves apparent.
The earlier analysis of the effect of changes in the eccentricity of the Earth's orbit was somewhat "descriptive" in style and, in particular, assumed that the eccentricity will continually decrease with time. However, although the eccentricity is indeed currently decreasing, this has been so only since about 10,000BC: before that it was increasing. After about 30,000AD it will again begin to increase and so over the timescale of my Saros Series investigations the eccentricity displays "pseudo-periodic" behaviour (see figure to the right). Given that the maximum in this graph is at the same period in time as the hump in Fig.15 it is tempting to ask what effect the variation in eccentricity might have on the numbers of eclipses in series at these distant epochs. To investigate this we shall have to move from a descriptive approach to one based on calculation - this is where the "indirect" effects of the eccentricity changes make themselves apparent.
The problem here is that, although the model used to calculate the eccentricity is valid for a very long time-period, the model used to calculate the motion of the Moon is not [by "model" I mean a set of mathematical expressions describing how the various parameters of, in this case, the Moon's orbit change over time]. If the position of the Moon cannot be accurately known it is not possible to guarantee that a predicted eclipse will actually happen, and so the number of eclipses in a given Saros series is likewise subject to doubt. If the starting number is not certain, it will clearly be difficult to distinguish any changes caused by the variation in eccentricity.
The time-period over which the expressions describing the lunar orbit are fully valid is relatively small (about +/-6000yrs from the current epoch), for two main reasons. Firstly, because the effects of the planets on the Moon cannot be calculated with perfect accuracy (as the various perturbations are all inter-dependent) and secondly because the expressions are, in part, derived from a process of curve-fitting rather than encapsulating any knowledge of actual orbital mechanics. Curve-fitting works by adjusting the various terms in the expressions until the result of using them to calculate lunar positions is a good match to the values calculated by numerical integration over a wide-enough range to be useful. Unfortunately, although the error in any calculation which uses these expressions outside their range of validity will start off reasonably small, there will come a point where the results suddenly become not just slightly in error but wildly so. This is known as the "exponential explosion" because it is caused by the presence in the expressions of high powers of the variables involved (cubes and fourth powers, typically) and the mathematical name for a power is "exponent". The explosion has nothing to do with orbital mechanics, but is purely a consequence of the limitations of the curve-fitting process. However, this problem means that, for example, the calculation of an accurate value for delta-gamma at distant epochs will be doomed to failure as its value depends on the difference between the synodic and draconic periods which are themselves calculated from expressions of the type we have been discussing.
Even the results produced by the Solex program eventually become potentially unreliable due to small uncertainties in the calculation parameters. Errors tend to keep building up slowly though, rather than suddenly becoming dominant, so even at distant epochs some reliance can be placed on the general trends shown by the calculations. I thus felt that there was some evidence to suggest that, while the variation in the eccentricity of the Earth's orbit clearly causes some of the changes in the shape and height of individual peaks in Fig.13 (as described in the previous section), the "pseudo-periodicity" of the variations may also produce a corresponding very long period variation in the average number of eclipses in a series. The underlying mechanism involved here is quite complex, and took me a lot of research to discover! I have placed the full explanation on another of my "maths" pages, so click here if you are interested. Otherwise, you'll just have to accept that it turns out that the way the eccentricity changes is [indirectly] built into the expressions which define the Moon's motion: it therefore has an influence on the synodic and draconic periods and hence delta-gamma. The way it is built in is slightly approximate, but I was able to quantify this approximation and thus determine the time-period over which the expressions for the motion of the Moon are "accurate enough" for the current purpose i.e. can be used to give sufficiently accurate values for the synodic and draconic periods, even if not for the precise position of the Moon, while also avoiding the exponential explosion. This has great consequences for the "believability" of my results.
Having determined that the eccentricity did affect the numbers, I used the expressions for the motion of the Moon to calculate delta-gamma and thus the number of eclipses over a long time-period. When plotting them on a graph, however, I was now able to indicate the results I had confidence in by showing the accuracy limits: this ensured I was not "over-interpreting" the results.
I was able to demonstrate the above phenomena by plotting the values I had calculated for the mean number of eclipses per Saros series, firstly using the standard expressions for the Moon's orbit. The result is shown as Fig.16. The vertical lines indicate the accuracy limits I derived from my study of the eccentricity variations (mentioned in the previous sub-section). These encompass all of Fig.15 with the exception of the far-right section (which runs to 19,000AD whereas I claim accuracy only to 14,000AD). This section is, however, the "least interesting" bit of Fig.15! It can be seen that the shape of the graph between the limit lines is a pretty good match to that of the baseline level of Fig.15. The values are slightly different because Fig.15 shows actual numbers but Fig.16 shows a mean value, which will clearly lie somewhere between the maximum and minimum level (as indeed it does).
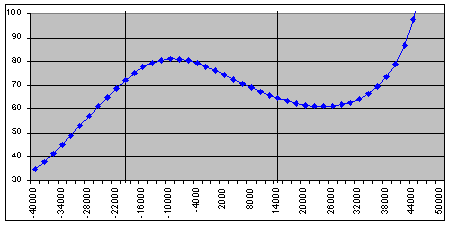 Fig.16
Fig.16
Next, I used expressions for the Moon's orbit for which a greater range of accuracy is claimed but which are purely mathematical in nature i.e. the terms in the expressions do not directly relate to physical phenomena [these expressions are described in the "maths" page mentioned above]. Click/tap on Fig.16. to see the result. While the behaviour of this graph is clearly totally different in the distant past and rather different in the far future (as a result of the more dramatic "exponential explosion" effect caused by the extra terms in the expressions used to create it) it is interesting to note that a) between the limits, the graph is very similar to that produced by the standard expressions [the major difference is an "uplift" to the right of the left-most limit line], and b) the shape of the graph is a very good match to the baseline level of Fig.15 (and better than the original one). The graph below, with the appropriate section of the graph overlaid onto Fig.15, shows this match clearly - the agreement is really quite convincing.
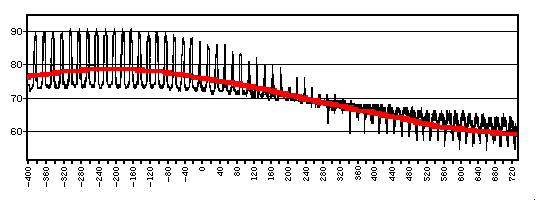
This closer agreement confirms the statement that the new expressions are more accurate, but the fact that the graphs are similar in shape supports my assertion that as long as one keeps within the limits the results given by the standard expressions are "good enough" for the purposes we are considering. I thus feel confident in saying that the overall shape of Fig.15 is indeed due to the effect of the changing eccentricity of the Earth's orbit.
Finally, I extended the work I did to explore the accuracy limits by determining which of the terms in the expressions for the Moon's motion were there to account for tidal, planetary and eccentricity effects [this additional work is also detailed in the "maths" page mentioned above]. I then set the tidal terms to zero and repeated my calculations. This showed that the "slope" of the graph with no tidal effect [pink] was greater than that with a tidal effect [blue] - see below. It can be seen that with no tidal effect the number of eclipses per series increases faster and to a higher maximum as we go back in time compared to the "with tides" situation, in agreement with Luca Quaglia's graph.
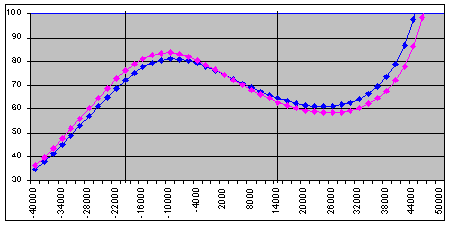 Fig.17
Fig.17
The model below allows all combinations of tidal, planetary and eccentricity effects to be demonstrated. Starting from a position of no perturbations (when the average number of eclipses per series is, unsurprisingly, constant) you can add (+) or remove (-) each effect independently by clicking or tapping on the appropriate button(s) and see what the result is.
|
|
The following observations should be noted:-
And so, it is at last time to discuss the very complicated topic of the consequences of the new Moon and apogee/perigee cycles being in step only every third Inex. Take a deep breath and fasten your seatbelt securely!
Given that the Moon's orbit is considerably more eccentric than that of the Earth, one should not be surprised that its varying distance and speed should have an influence on the number and type of eclipses in a Saros series. In fact, these variations have many effects, some because of the varying distance and some because of the varying speed.
Firstly, because a "distant" Moon will generally result in an annular eclipse and a "near" Moon a total eclipse (due to the variation in its apparent size), series whose eclipses have the middle part of their sequences spanning lunar apogee will consist of annular eclipses while those where lunar perigee is spanned will consist of total eclipses. More interestingly, where the apogee/perigee status changes significantly as the series progresses the type of eclipse can change from annular to total or vice-versa. The change-over from annular to total happens when the apparent size of the Moon just exceeds that of the Sun. However, this point is (on average) about +/-41/4 days away from alignment with perigee rather than half-way between apogee and perigee (67/8 days) as one might have expected. This is because, with the current orbit of the Moon, its apparent size is less than that of even the smallest-sized Sun until the Moon itself is larger than average. The apparent size of the Moon increases as it moves from apogee towards perigee, and so the point of size equality will be closer to perigee than the half-way point. In fact, [as I will show very near the end of this major section] the furthest from perigee the Moon can be and the eclipse still be total is about 61/2 days: this requires the Earth to be at aphelion however, when the apparent size of the Sun is at its smallest. With the Earth at perihelion the maximum distance is only about 22/3 days. [Note that 41/4 days is not the simple mean of these two values because the distribution of high and low numbers is not symmetrical]. There are therefore more circumstances that could result in an annular eclipse rather than a total, which should mean that there are more annular eclipses than totals, and this is indeed true.
 The change from annular to total eclipses from series to series is perhaps the most obvious demonstration of the fact that the new Moon and apogee/perigee cycles are in step only every third Inex (i.e. every third Saros series). These sets of three are easily seen in the Saros-Inex Panorama (repeated at left) where distinctive red-red-blue and blue-blue-red patterns repeat every three series throughout the non-partial phases. A geometric way of looking at this is to imagine that eclipses from successive series are spaced by 120deg from each other round the Moon's orbit. If we say that apogee is at zero degrees and perigee at 180deg, if a particular Saros series has its non-partial eclipses spanning apogee (thus producing annular eclipses) it won't be until the third series afterwards that the same will be true again. The two series in between will span the intervals just before and just after perigee (i.e. at 120deg and 240deg) and hence will tend to produce eclipses which are either all total or (more often) a mixture of the two types. However, because even the "times 3" relationship is slightly inexact (it is in error by about 0.02 of an orbit), the patterns will slowly drift over time: in terms of the geometric representation this can be imagined as the eclipse points slowly moving round the orbit while staying 120deg from each other. This drift can be seen in the Panorama, where the overall shapes of the mainly-red and mainly-blue areas clearly repeat at regular intervals - note also the regular repetition of the patches of "yellow" (i.e. hybrid) eclipses. Visually, the periodicity of these repetitions is a little less than three "sawtooth periods": as this is about 19 Saros series (as already mentioned) the periodicity will be somewhat under 57 series. Multiplying the number of perigee-to-perigee lunar orbits in an Inex [the accurate value of which for the part of the Panorama in question (around 2000BC) is 383.6729] by numbers slightly less than 57 gives a result closest to "plus a third" for a periodicity of 54 series - 20718.3353.
The change from annular to total eclipses from series to series is perhaps the most obvious demonstration of the fact that the new Moon and apogee/perigee cycles are in step only every third Inex (i.e. every third Saros series). These sets of three are easily seen in the Saros-Inex Panorama (repeated at left) where distinctive red-red-blue and blue-blue-red patterns repeat every three series throughout the non-partial phases. A geometric way of looking at this is to imagine that eclipses from successive series are spaced by 120deg from each other round the Moon's orbit. If we say that apogee is at zero degrees and perigee at 180deg, if a particular Saros series has its non-partial eclipses spanning apogee (thus producing annular eclipses) it won't be until the third series afterwards that the same will be true again. The two series in between will span the intervals just before and just after perigee (i.e. at 120deg and 240deg) and hence will tend to produce eclipses which are either all total or (more often) a mixture of the two types. However, because even the "times 3" relationship is slightly inexact (it is in error by about 0.02 of an orbit), the patterns will slowly drift over time: in terms of the geometric representation this can be imagined as the eclipse points slowly moving round the orbit while staying 120deg from each other. This drift can be seen in the Panorama, where the overall shapes of the mainly-red and mainly-blue areas clearly repeat at regular intervals - note also the regular repetition of the patches of "yellow" (i.e. hybrid) eclipses. Visually, the periodicity of these repetitions is a little less than three "sawtooth periods": as this is about 19 Saros series (as already mentioned) the periodicity will be somewhat under 57 series. Multiplying the number of perigee-to-perigee lunar orbits in an Inex [the accurate value of which for the part of the Panorama in question (around 2000BC) is 383.6729] by numbers slightly less than 57 gives a result closest to "plus a third" for a periodicity of 54 series - 20718.3353.
| The relevance of the "plus a third" is, of course, that after a whole number of rotations plus a third the positions of eclipses round the orbit will, effectively, be back to where they started as each will have moved by exactly the spacing between them - one-third of an orbit, or 120deg. This explains the repetition of the eclipse patterns at the periodicity calculated. It also explains the shape of the "sawtooth" patterns of the Saros-Inex Panorama. Referring back to Fig.2a again (the top part of which is repeated on the right), it is clear that although the "mini-teeth" on each larger sawtooth are made up of repeating patterns of sets of three the actual pattern on each sawtooth varies. We now see that this is because the apogee/perigee repeat periodicity (54) is not evenly divisible by the Panorama repetition period (19): this will result in a shift in the apogee/perigee situation at the start of each sawtooth and thus, as we shall see below, a different set of eclipse patterns. |  |
Having established the fact that Saros series come in sets of three, the obvious next question is - so what? Well, the difference between the eclipses forming the first, second and third members of each trio is the apogee/perigee status of the Moon. The apogee/perigee status causes not just the large-scale changes from total to annular mentioned above but also more subtle eclipse-by-eclipse changes due to variations in delta-gamma: its maximum value can change by almost +/-10%, for example. Neither I nor Jean Meeus have been able to determine exactly what mechanism causes these changes but I suspect it is a combination of many factors such as variations in orbit speed, the changing longitude difference between the true and mean nodes and the variation in length of the various lunar periods from cycle to cycle (caused by perturbations from the Earth and Sun). Suffice it to say that, pretty consistently, proximity to apogee increases maximum delta-gamma and proximity to perigee decreases it. [The effect also exists for minimum delta-gamma but is neither as great nor as consistent as at maximum, again for reasons I do not fully understand].
It should thus be clear that the delta-gamma curves for the three Saros series that are members of an "Inex Triple" are quite likely to be considerably different from one another. Fig.18 shows this well. At the first peak, the eclipses of Saros series 33 were taking place just one day before lunar perigee, those of Saros 34 were two days past apogee and those of Saros 35 were four and a half days after perigee. It can be seen that the maximum values of delta-gamma attained are exactly in accord with the "apogee larger/perigee smaller" rule. Similarly, at the second peak the eclipses of Saros series 33 were taking place four days after apogee, those of Saros 34 were six days before apogee and those of Saros 35 were one day before perigee - the delta-gamma values are again in agreement. Note that because the actual apogee and perigee distances vary considerably from orbit to orbit (apogee much more so than perigee) the variations in delta-gamma caused by apogee/perigee will themselves vary somewhat according to the exact distance at a given eclipse: strict graph-to-graph comparisons are thus not possible, only general trends. This is enough to establish solid conclusions though.
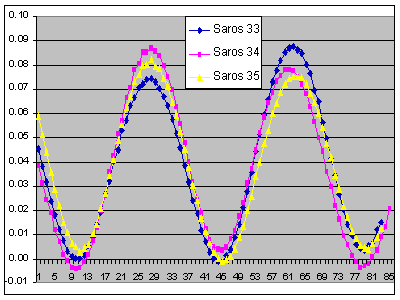 Fig.18
Fig.18
Changes in delta-gamma will have an appreciable effect on the gamma curve for a Saros series, as is shown by the aphelion/perihelion effect that gives these curves their shape in the first place. A change in maximum delta-gamma will have a particularly noticeable effect, as this is what determines the vertical distance between the plateaux in the graph and thus, as noted above, most of the major features of the gamma graphs. This can be seen in Fig.19, which gives the gamma curves for the same three Saros series as Fig.18.
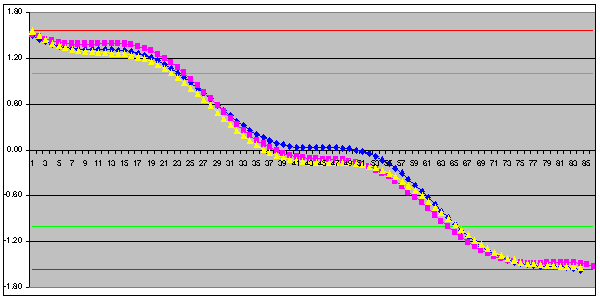 Fig.19
Fig.19
Observe that on the first plateau, where Fig.18 tells us that series 33 has a zero delta-gamma, series 34 actually has a negative value, and series 35 has a small positive value, the gamma curve is flat for series 33 (blue), rises slightly for series 34 (pink) and falls slightly for series 35 (yellow). During the first steep section, series 34 falls the fastest followed by series 35 then series 33 i.e. in the order of their delta-gamma values at the first maximum. Similarly, the series with by far the largest delta-gamma at the second maximum, series 33, falls much the fastest during the second steep section. Many other correspondences can also be found. Those who are catching on fast will realise that this behaviour is why I showed only every third Saros series in Fig.10 above! To have shown all the series would have confused the effect I was wishing to show with the AP effect demonstrated here. It also explains the offset between series 34 and 52 observed in Fig.10a: the apogee/perigee pattern for series 52 is more similar to that for series 33 than series 34 and so, in the same way as in Fig.19, the different rates of fall of the steep sections produce an offset in the central sections of the graphs.
It does not take much imagination to realise that if a trio of series had a plateau close to the no eclipse/partial eclipse or partial/non-partial border, a change to either the slope of the plateau or the angle of the steep section could easily move the plateau to one side of the border or the other, thus changing the number of eclipses in the phases and quite possibly the total number of eclipses in a series overall. This is in fact shown to a small extent in Fig.19. Observe that because the graph for series 34 (pink) falls less steeply in the last third of the graph (and from a slightly higher initial level) and has a final plateau that rises slightly before finally falling, it can carry on for more eclipses before crossing the no-eclipse line. This results in it having a greater number of closing partials (23) as compared to both series 33 and 35 (19), and 2 more eclipses overall.
The fact that the AP effect can alter the distribution and number of eclipses in a Saros series has two main consequences. Firstly, the effect can produce series whose distribution of eclipses is anomalous as compared to its neighbours. This is, in fact, the reason that Fig.1 and Fig.4 (for example) are not smooth graphs - the "spikes" are caused by the imperfect overlap of graphs produced by the three sets of series. Re-plotting using just the first, second or third members of each Inex Triple removes these anomalies to give a much smoother graph. This is shown by Fig.20, which is a re-plot of Fig.1 produced in this way [click/tap to move from graph to graph and then see a composite of all three sequences]. Observe that the third peak in the top row is almost exactly the same for each graph and so will produce a smooth result when all three are added together, but that the peaks in fifth place are definitely offset and so give an "M" shaped result when added: this is exactly what we see in Fig.1. [a small version of which is shown to the right of Fig.20.]
 | 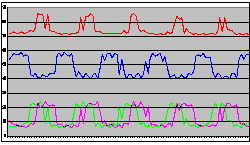 |
| Fig.20 |
While the first consequence of the AP effect relates to short-term changes in delta-gamma between adjacent series, the second consequence relates to long-term changes, when the ability of the effect to either add to or subtract from the otherwise constant increase in delta-gamma over time can potentially bring forward or hold back the transition to the "constant total" and/or "diminished total" regimes described above. Indeed, the extent to which it can modify delta-gamma can produce these regimes even when they should not happen - as we shall see very shortly.
Note that, in the composite, there is a tendency for sections of two graphs to be very similar while the third is quite different (though which are similar and which different changes along the sequence). This can also be seen in the delta-gamma graphs and reflects the fact that if, for example, an eclipse in one series aligns with lunar apogee the corresponding eclipses in the series just before and just after will each align one-third of an orbit away from lunar perigee: one before perigee and one after. These two eclipses will thus be very similar, and different from the first one. As the eclipse points move round the Moon's orbit, which one is the "odd man out" will change, as seen on the graphs.
Note also that, while I have already remarked that the Moon-Earth distance is the major factor in determining whether an eclipse will be total or annular, in a different context the fact that the Moon's speed is determined by its distance from the Earth is almost as important. Its orbital speed is critical in determining the precise moment of eclipse and so the variability of the distance, and thus the speed, from one Inex to the next means that successive eclipses nominally separated by one Inex period will actually have quite a spread of time differences between them. For example, the interval between the seven "Inex-period" eclipses between 10th October 1912 and 11th June 2086 varies from 10571.48 to 10572.66 days - a spread of 1.18 days. This is much greater than the spread of the Saros period, which is only 0.055 days over the three centuries from 1901 to 2200. While one might just dismiss this issue by saying that the Inex is thus no good for actually predicting eclipses, the problem is that the Sun-Moon-Earth geometry keeps changing during this time and so any variation in the actual moment of eclipse will affect the gamma value of the eclipse when it does occur. This clearly has serious repercussions for any process that depends on gamma (and delta-gamma), such as the variability of number of eclipses in a Saros series. Just one more factor to add to the many [apparently] random variations that complicate any attempt at a detailed analysis!
We saw above that plotting using only one of the set of three Saros series in an Inex Triple results in much clearer graphs with no "spikes". It is instructive to apply this principle to the graph of past, present and future Saros series, given as Fig.13 above.
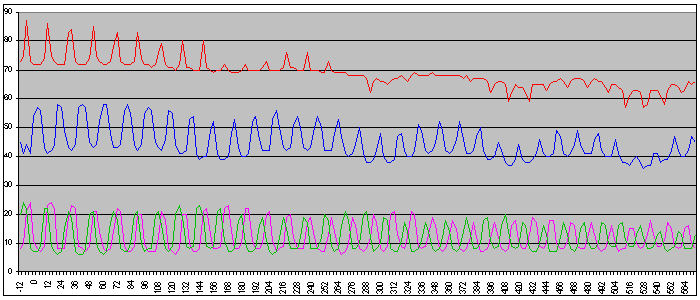 Fig.21
Fig.21
Fig.21 is Fig.13 re-plotted using only data from series -12,-9,-6,-3,0,3,6,9 etc. There are two obvious differences as compared to Fig.13. There is, as we would have hoped, an absence of spikes (as per Fig.17 compared to Fig.1) but, more importantly for our present discussion, some of the structure in the graph of total number of eclipses in a series (in red) present in Fig.13 is missing here. There are now several sections where it hardly moves from the baseline, to give a clear "constant totals" effect as previously predicted. Less obvious, perhaps, is the fact that the graphs now have a periodicity - most clearly shown in the slow up-and-down wave of the graph of non-partials (blue). It is a little difficult to give an exact frequency for this periodicity, as the graphs are not perfectly regular, but I tried to get a good approximation by deriving the mid-line of the non-partials graph and plotting the distance between its troughs and crests. I found that a good mid-line was produced by taking 6-point averages six points apart - there is a logic to this, as of course many peaks of the graph are about 18 or 19 series apart so if you plot every third series you will end up with 6 points per peak. Click/tap on the diagram to see the resultant "wave".
The result of this exercise is that the distance between the wave crests starts out at about 150 series on the left and then steadily reduces to about 100 series on the right. Can we deduce anything from this? Indeed we can! You will recollect that the periodicity of the patterns seen in the Saros-Inex Panorama was 54 series, which was shown to be the time taken for the eclipse points to drift 120deg round the Moon's orbit. Having plotted only every third series, we are here considering only one of the three eclipse points however, and so the relevant periodicity will be for a full 360deg rotation, which is clearly 162 series. This was at an epoch of 2000BC, however (i.e. Saros series numbers around 30). Repeating the calculations for the epochs for which Meeus gives highly accurate values for the synodic, anomalistic and draconic months (in Morsels III) shows us that around 0AD the corresponding value is 153, at 2000AD it is 147 and at 4000AD it is 138. Plotting these values against their corresponding Saros series numbers gives a pretty straight line, as one might expect. More interestingly, however, the graph produced when the "wave" periods are plotted against the mean of the Saros series numbers constituting each wave is almost exactly the same line - see Fig.22 (the blue data is from the "waves", the pink is from the calculations).
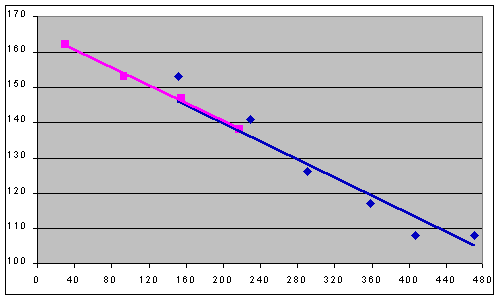 Fig.22
Fig.22
This close correspondence makes it certain that the waves are caused by the AP effect. This conclusion is reinforced if one looks at the graphs corresponding to Fig.21 for series -11,-8,-5,-2,1,4,7,10... and -10,-7,-4,-1,2,5,8,11... They are similar in shape to that for series -12,-9,-6,-3,0,3,6,9... but the waves are shifted along the x-axis. This can be seen if you click/tap on the repeat of Fig.21 below to show each of the graphs in turn.

It is clear that the three graphs follow on from each other to give a complete flowing sequence, consistent with the AP effects producing them being 120deg out-of-phase due to the Inex "sets of three" phenomenon [this is particularly clear if you click/tap quite rapidly]. It is also now apparent why Fig.13 essentially shows only "peaks & troughs", not flat areas. When all the graphs from Fig.21 are added together to give Fig.13, a flat area from one graph will usually be over-ridden by the structure from the other two and so will not remain visible. It is only by separating the Inex Triples that the behaviours predicted by the early analyses can be fully seen.
Now we know that the waves are caused by the AP effect we can begin to correlate the variations in delta-gamma that we know the effect will cause with the changes in the total number of eclipses in a Saros series. The best graph to take as our test-case is that of the non-partials, as the "waviness" is most obvious here. It is clear that when the mean value of this graph falls, the total number of eclipses is smaller than it would otherwise have been i.e. a run of upward peaks can be changed into a relatively flat section and a flat section can acquire "downwards peaks". The first effect is particularly dramatic in the case of the set of series starting with -13 (the third member of Fig.21). Here, the upward peaks that should have existed at the left-hand side for Saros series 92, 110 and 128 are hardly visible. In these cases, the "inherent" value of delta-gamma is certainly not large enough to produce the constant-total regime and so it can only be the effect of the apogee/perigee status that is causing this to happen. Further investigation proves this to be the case.
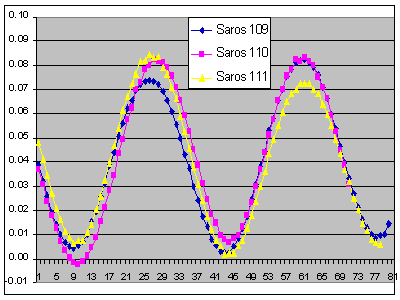 Fig.23
Fig.23
Consider Fig.24 - a plot of delta-gamma for the Saros series close to the middle "missing peak". In the case of series 110, the values of delta-gamma at each peak are slightly greater than average while that in the trough is considerably greater. The earlier discussion about the AP effect would thus indicate that the eclipses near to each peak were somewhat closer to lunar apogee than perigee and that those in the trough were very close to apogee. This is confirmed by the data: the eclipse at the first peak is 4days 18hrs after apogee, that in the trough is just 12hrs after apogee and that at the second peak is 4days 1hr before apogee [The eclipse point moves "backwards" because the perigee-to-perigee version of the Saros period is longer than the new Moon-to-new Moon version]. This means that all the non-partial eclipses will be much closer to apogee than to perigee and so should all be annular: this is confirmed by the NASA data. I shall leave a similar analysis of series 109 and 111 as "an exercise for the student"!
More importantly for our present investigation, however, the very different delta-gamma history of series 110 as compared to series 109 & 111 (whose graphs are in fact the inverse of each other - low-low-high and high-low-low respectively) means that its gamma-curve is also very different, as shown by Fig.24.
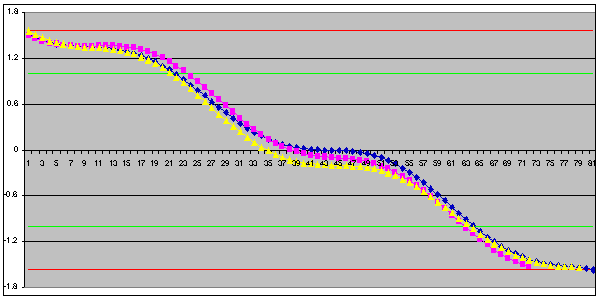 Fig.24
Fig.24
The greater delta-gamma values of series 110 (pink line) throughout the central part of its "life" cause its graph to descend more steeply than both the other series, with the eventual result that it crosses the lower eclipse-limit line much sooner than they do. This means it has many fewer closing partials and so, despite being potentially a "three plateau" curve, because the final plateau has been pushed below the eclipse limit by the steeper descent its overall total of eclipses is considerably smaller than either series 109 or 111. The numbers are 21/43/17=81, 23/39/10=72 and 21/42/16=79 in fact. Note that the inverse-symmetry of the delta-gamma curves for series 109 and 111 is reflected in their gamma-curves: though diverging in the middle section they eventually end up on top of one-another.
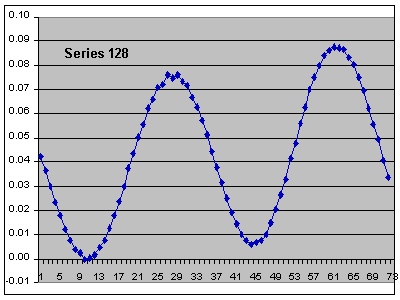
The situation for series 92 and 128 is different from that for series 110, but with the same final result. The case of series 128 is the clearest. Instead of having larger delta-gamma values throughout the middle of its life, this series has much greater values near the second peak (due to the very close proximity of eclipses in this area to lunar apogee) - see diagram at left. This results in the second steep section being much steeper than usual, pushing the lowest plateau beyond the eclipse limit almost before it has begun to flatten out - see below.
Series 92 is the exact reverse - it has very large delta-gamma values at the first peak, pushing the middle plateau so far down the graph that even though the second steep section descends less steeply than average there is still insufficient room for a final plateau to form. Click/tap on either diagram to see the graphs for the two series alternately.
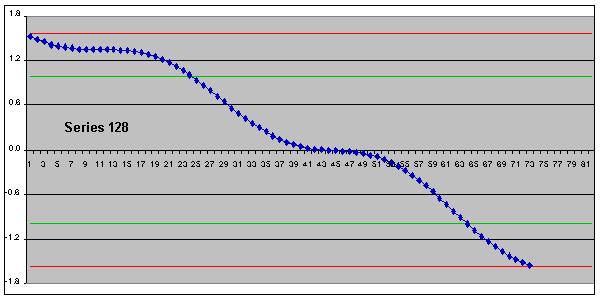
All three series thus have a smaller number of eclipses than expected because of their unusually low numbers of closing partials, this being caused by the large delta-gamma values produced when a series spans lunar apogee. This process will only come to an end when the eclipses in a series are no longer close to apogee, which can most easily be spotted by noting when hybrid eclipses appear (i.e. cases on the annular/total borderline). In fact, series 71 is "29A 3H 9T", series 92 is "40A", series 110 is "39A" and series 128 is "4T 4H 32A". The progression is (as ever!) slightly upset by the repetition period of the peaks (19 series) not being divisible by three, and by the fact that there are no "entirely annular" series around no. 128, but even so the conclusion is clear. Before and after this list the AP effect will probably not be strong enough to cause sufficient loss of eclipses overall and hence it will only be in this range that peaks disappear - exactly as found in Fig.18. Similar ranges will, of course, occur for the members of the other two Inex Triples, resulting in them having their own "peakless" sectors - the one for the first member of Fig.18 is quite clear [series 165, 183 & 201] but that for the second member merges with its "inherently flat" section and so is not so obvious.
We have seen how consistently large values of delta-gamma caused by the proximity of eclipses to lunar apogee can convert a potentially "large total numbers" series into an "ordinary" one, thereby removing a peak from the overall data. Making peaks disappear is not the only trick of which the AP effect is capable, however! It can also make them appear, but in a downwards direction. This is most clearly seen on the right-hand side of each member of Fig.18, where dips are present whenever the non-partials graph has a trough. It must also be the explanation for the curious single downward features seen, for example, between series 288 and 300 on the first member of Fig.18, as they are also aligned with troughs in the non-partials graph. I cannot present any detailed analysis to prove this, unfortunately, as data is only available from NASA for series -13 to 190. However, the Saros-Inex Panorama shows that each of the dips is associated with series consisting of only annular (i.e. apogee-type) non-partial eclipses. I thus think it is clear that the creation of these dips must come about in a similar way to the deletion of peaks - if this is not what is happening then the association with the AP effect-generated waves would be very difficult to explain.
I suggested above that the AP effect would only have a strong influence for eclipses where the Moon was reasonably close to apogee. Taking the example of series 110 above, while the eclipses close to the central minimum occur very close to apogee and have significantly increased values of delta-gamma, the eclipses at its two crests occur a little more than 4 days from apogee and have "normal" values of delta-gamma: 4 days away is thus clearly not "reasonably close to apogee". Looking at the numbers for other series confirms the conclusion - the effect disappears rather abruptly around the +/-3.75 days mark. Now, from Fig.19, at the epoch we have been considering there are about 153 series between the times the "point of eclipse" is close to apogee. The interval between the missing peaks is 18 series, which is 11.8% of a full circuit or 3.24 days. Also, as I stated much earlier in this article, the point closest to apogee moves by 2.82 degrees per eclipse in a series. Given that the number of eclipses from a crest to a trough in a delta-gamma graph is about 18, the total movement will be 50.8deg, or 14.1% of a full circle. A perigee-to-perigee lunar orbit takes 27.55days, so this represents 3.9 days (hence the ~4day difference between crest and trough for series 110). In other words, by these calculations the shift between two series exhibiting missing peaks ought to be less than the limit for the disappearance of the AP effect but that from trough to crest in the same series should be greater than the limit. This confirms the finding that series 109 only has a strong effect for its central trough and shows why the missing peaks come in three's - if a given series is centred on apogee there is just room for there to be one more on each side of it which feels the effect, but only one more.
It also gives an insight into the appearance of hybrid eclipses. If the crests of the series centred on apogee are about +/-4 days from apogee, those most far from apogee in the series on each side will be 4+3.2 = 7.2 days away. To determine whether this result is significant I had to find the range of the annular/total changeover point. To do this I plotted the distance from perigee (in days) of the middle eclipse in every run of hybrid eclipses [if any] for every Saros series from 0 to 180. I then determined the "average largest" and "average smallest" distances when a hybrid eclipse is still just possible (there is a range because of the variable apparent size of the Sun). You can read the full exploration of this calculation by clicking here, but the answer is that the least distance from apogee that a hybrid eclipse can occur is 7.3 days: this requires the Sun to be small however. But wait! By definition, the eclipse dates at a crest must be around aphelion in order that delta-gamma be large and of course the Sun is indeed at its smallest at aphelion. In other words, eclipses near to the "more distant" crest of the series before and after the central one will be close to the annular/hybrid limit and will thus need only a slight variation to be hybrid rather than annular.
Anything involving the orbit of the Moon never exactly accords with theory of course, but real-life is at least basically in line with these conclusions. For each series mentioned above (71, 92, 110, 128), I calculated the crest-to-trough intervals and the shift between similar features from series to series and the results were in reasonable agreement with the above calculations. The average values were in fact 4.1 and 3.7 days respectively, though with a range of about +/-0.8 in each case: the first number was always greater than the second, however. The reason that series 128 has a few total eclipses at its start seems to be because series 110 is not exactly centred on apogee - it is at "A+12hrs" in fact. This means that the first crest of series 128 is further from apogee than it "should be" and so crosses the annular/hybrid limit sufficiently to produce not just hybrids but totals as well. Conversely, the last crest of series 92 is less far from apogee than average and so doesn't get close to producing even hybrid eclipses let alone totals.
There is a further reason why a series might be "further from apogee than it should be", to do with the amount that the point of eclipse moves from peak to peak. Given that there are three independent sets of series (what I have called the "Inex Triples") and that just three series in a row from a given set can be near enough to apogee for the AP effect to delete a peak, if the shift between such series was exactly one-ninth of a perigee-to-perigee lunar orbit then each of them would neatly move into position in sequence. Now one-ninth of an orbit is 27.55/9=3.06 days but, as we saw above, the shift is actually 3.24 days: a difference of 0.18 days. In other words, each peak moves just a bit too far for all of them to nicely align with apogee. The consequence is that, eventually, one of the series might well be too far away from apogee for the AP effect to have an influence and so there should be a "missing" missing peak (if you see what I mean!). Let's consider the next peak in the above series - number 146.
Given that series 128 had a few totals in its sequence, one would expect 146 to have even more, and indeed it does - its sequence is 13T 4H 24A. Its final crest is just on the limit for the AP effect to have an influence though (A+3d15h) so it does lose a few closing partials to give a total of 76. Series 128 is thus clearly the last peak to be fully missing for this Inex Triple. The first one for the next Triple should therefore be series 147, but this has 80 eclipses! Have we found our "missing missing peak"? Well - yes and no!
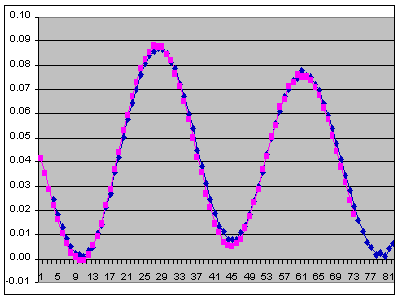 As one might expect, series 147 has an almost identical delta-gamma curve to series 92 because they are both the first member of a new sequence: see the diagram to the left (series 92 in pink, series 147 in blue). In addition, all its non-partial eclipses are annular - it should therefore indeed be a "missing peak". The reason it is not is, however, nothing to do with slipping too far past apogee: in fact, the first crest of series 92 is at A+0d23h and that for series 147 is at A+1d10h, still well within the AP effect "window" [Note that the difference (11hrs) is pretty close to 0.18 days * 3 (=13hrs) as would be predicted from the "slippage" calculation above]. In truth, the difference of 0.18 days per peak is rather too small to have a significant effect after only a few peaks. The reason is rather that, because we have moved from one Inex Triple to the next, the calendar date of the first eclipse in the series has changed. Series 92, 110 and 128 started in late August but series 147 (and indeed 165 & 183) begin about 2 months later. This difference is crucial because, due to the aphelion/perihelion effect, the nearer we get to January the smaller the value of delta-gamma [the small variation in the actual date of perihelion over the time-span of the beginning of these series will not be significant]. So although the overall shape of the curves is the same, the initial values of delta-gamma for series 147 are actually much smaller than those for series 92. I had to allow for this by offsetting the curve for series 147 when plotting the graphs: click or tap on the diagram to plot both curves without any offset, when the difference will be very clear. The gamma-value graph for series 147 will therefore start "further up the page" than that for the eclipses of the previous Inex Triple and thus will be less susceptible to having its third plateau pushed over the eclipse limit by the AP effect: see Fig.25 below. It will lose a few opening partials (because its first plateau occurs soon after the series starts) but will retain all its closing partials to give a larger total overall. Once the new sequence is established, however, the AP effect can begin to exercise its influence again: series 165 is almost identical to series 110 and is a "missing peak", as is series 183. I cannot plot the graphs for series 183 unfortunately (the full NASA data only goes up to series 180) but it would seem likely it is an analogue of series 128 as it has a very similar eclipse sequence.
As one might expect, series 147 has an almost identical delta-gamma curve to series 92 because they are both the first member of a new sequence: see the diagram to the left (series 92 in pink, series 147 in blue). In addition, all its non-partial eclipses are annular - it should therefore indeed be a "missing peak". The reason it is not is, however, nothing to do with slipping too far past apogee: in fact, the first crest of series 92 is at A+0d23h and that for series 147 is at A+1d10h, still well within the AP effect "window" [Note that the difference (11hrs) is pretty close to 0.18 days * 3 (=13hrs) as would be predicted from the "slippage" calculation above]. In truth, the difference of 0.18 days per peak is rather too small to have a significant effect after only a few peaks. The reason is rather that, because we have moved from one Inex Triple to the next, the calendar date of the first eclipse in the series has changed. Series 92, 110 and 128 started in late August but series 147 (and indeed 165 & 183) begin about 2 months later. This difference is crucial because, due to the aphelion/perihelion effect, the nearer we get to January the smaller the value of delta-gamma [the small variation in the actual date of perihelion over the time-span of the beginning of these series will not be significant]. So although the overall shape of the curves is the same, the initial values of delta-gamma for series 147 are actually much smaller than those for series 92. I had to allow for this by offsetting the curve for series 147 when plotting the graphs: click or tap on the diagram to plot both curves without any offset, when the difference will be very clear. The gamma-value graph for series 147 will therefore start "further up the page" than that for the eclipses of the previous Inex Triple and thus will be less susceptible to having its third plateau pushed over the eclipse limit by the AP effect: see Fig.25 below. It will lose a few opening partials (because its first plateau occurs soon after the series starts) but will retain all its closing partials to give a larger total overall. Once the new sequence is established, however, the AP effect can begin to exercise its influence again: series 165 is almost identical to series 110 and is a "missing peak", as is series 183. I cannot plot the graphs for series 183 unfortunately (the full NASA data only goes up to series 180) but it would seem likely it is an analogue of series 128 as it has a very similar eclipse sequence.
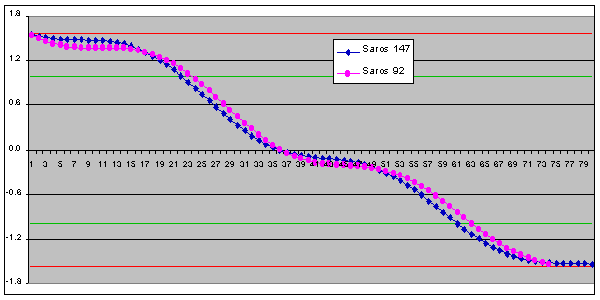 Fig.25
Fig.25
The above analysis is an excellent example of a relatively small change in initial conditions having a very pronounced effect on the number of eclipses in a series. This sensitivity is the reason that the AP effect exists at all, and is also the reason that the small changes in delta-gamma and the eccentricity of the Earth's orbit as the series number increases can result in very large changes in the number of eclipses in a series, as seen in such as Fig.13.
A fairly obvious question arises at this point however - if any series with a pronounced AP effect should result in a missing peak (series start-date permitting!), how come there are none missing before the sequence analysed above? The answer seems to lie in the fact that the mean value of delta-gamma is inherently smaller for "earlier" series. This will mean that the vertical distance between two plateaux on the gamma value graph is also smaller for these series and so it will not be possible for the variation in delta-gamma caused by the AP effect to shift a plateau over the eclipse limit and thus remove a peak. I showed earlier that the increase in delta-gamma over time eventually produces the "constant value" regime where there are no peaks at all. Just before this happens, the AP effect can supply the extra amount of delta-gamma and thus act to bring forward this regime for some series, producing missing peaks. Similarly, it will also act on later series to bring forward the "dips not peaks" regime, to give the anomalous "early" dips observed in Fig.18.
And just in case anyone has been looking at the NASA list of Saros series and noticed that series 15 and 31 both have low numbers of eclipses within a run of series having high numbers of eclipses i.e. they are apparently "missing peak" series, I'm afraid I have to disappoint you. I mentioned just above that, due to delta-gamma being inherently smaller, the plateaux in early series are closer together. This means that it may possible for two successive series in each Inex Triple to fit three plateaux between the eclipse limits despite the "right and down" shift we have observed many times in this article. This does, however, require the first plateau to be in the correct place initially. In the case of series 30 and 32, for example, the plateau is just below the eclipse limit, leaving enough "headroom" for series 33 and 35 to also have three plateaux. All these series thus have large numbers of eclipses. Series 31, however, has its first plateau just above the limit so, although there is thereby plenty of room for series 34 to have three plateaux, series 31 only has two plateaux and thus has a much smaller number of eclipses. So, while series 15 and 31 are, in a sense, "missing peak" series they are so for quite different reasons - they are not victims of the AP effect.
And finally! I have, at many places throughout this analysis, said that the periodicity of the Saros-Inex Panorama is "about 19 series". Although this statement can easily be verified simply by counting up the series between each "sawtooth", I felt I had to derive a reason for the number 19. This proved to be surprisingly difficult!
My first thought was that it had something to do with the fact that a Saros interval is 19 "eclipse years" - the periods of 346.62days after which a given node of the Moon's orbit re-aligns with the Earth-Sun line. However, given that the actual periodicity starts out at 20 or more for series with large negative Saros numbers and decreases to 15 or 16 for very large Saros numbers (far into the future!) but that the eclipse year will vary only very slightly over time (as the lunar orbit periods will change by only an extremely small amount) I felt this could not be the underlying cause. The key turned out to be the reason the Panorama has a sawtooth structure at all: the variation of delta-gamma caused by the aphelion/perihelion effect. Each "tooth" occurs when delta-gamma is reduced to almost zero, which happens at the time of perihelion. It therefore follows that the time interval between the teeth must be a whole number of perihelion-to-perihelion (or "anomalistic") years. There are only a small number of combinations of Inex and Saros intervals which will ensure this, which results in the particular periodicity seen. As ever, things are not quite this simple however so once again I have placed the detailed calculations on a "maths" page - click here to read all about it.
The outcome of these calculations is that one of the best combinations of Inex and Saros intervals to result in a whole number of calendar years is 19 Inex + 2 Saros. This sum is the origin of the well-known Tetradia period of 586yrs first discovered in connection with sequences of four successive lunar eclipses, called a Lunar Tetrad (hence the name). Tetrads are caused by an "aphelion/perihelion effect" similar to that which causes the variation in delta-gamma responsible for the change of numbers of eclipses in a Saros series, so it is perhaps not surprising that they might have a similar underlying periodicity. However, while the "by definition" Tetradia is 19I+2S, which equals 586 simple calendar years plus just 28.6hrs, the "real" value differs from this due to two factors - a) the anomalistic year is slightly longer than the simple calendar year, and b) there is a steady decrease in the "I" and "S" values over time (as shown in the full calculations page linked to just above). The current value of the Tetradia is about 565 calendar years, which those who have read the "Tricky Stuff" page of my article on solar eclipse patterns will recognise as the period of such other things as the distribution of years with five total solar eclipses. It is not entirely clear why the periodicity should exist at all, but the best theory relates it to a slight oscillation in the Earth's orbital position caused by one of the many subtle interactions between Sun, Earth and Moon.
The original study of Lunar Tetrads used limited historic lunar eclipse data centred around the year 479AD. The periodicity was measured as 586yrs using this data, hence the original definition of the Tetradia period (supported by the fact that, as indicated above, this version of the Tetradia is also an "eclipse cycle" i.e. an interval after which many aspects of eclipses will repeat almost exactly). Later work using more datasets showed that the period of the variability is in fact slowly decreasing with time according to the empirical formula P = 565.1 - 1.38*T, where T is the number of Julian centuries from the year 2000. This formula was then given a theoretical basis by a complex but rather impenetrable analysis as reported in the Addendum to chapter 21 of Mathematical Astronomy Morsels III by Jean Meeus, and thereby improved to P = 565.027 - 1.3149*T + 0.000288*T^2.
I was interested to see whether I could find any connection between this formula and the changes caused by the decrease in "I" and "S" values mentioned earlier. This rapidly became rather mathematical so, yet again, I've placed the calculations on a "maths" page - click here to check it out. The answer though is - yes, it can indeed be shown that the change in periodicity brought about by the decrease in I and S over time can be very closely modelled by the Tetradia formula, though this conclusion requires the formula to be extended to include terms in T^3. It can also be shown that the change in periodicity closely follows a relationship which would result in "whole number of years" values of the period, as required by my previous analysis. Whether there is a link between these two processes is, however, something I have been unable to determine.
At this point, therefore, my exploration of Saros series must end. However, anyone who has got this far is probably feeling a bit shell-shocked at this stage, so I shall finish by presenting a basic overview of my more important findings, expressed in terms of bunches of eclipses rather than delta-gamma as this concept is probably easier to visualise.
A solar eclipse can only occur if the position of full Moon is within a "window of opportunity" approximately 35deg wide centred on one or other of the nodes of the lunar orbit. This position will not be constant, however, as the "full-Moon" and "node-to-node" versions of the Saros interval are slightly different, which slowly moves the position through the window. However, when an eclipse occurs at perihelion the Earth is moving faster than average, and so the Moon has to spend a somewhat greater time getting to the position required for an eclipse. This lengthens the "full Moon" version of the Saros interval and so makes it more nearly equal to the slightly longer "node-to-node" Saros interval, which reduces the amount that the eclipse position moves through the window of opportunity. This causes eclipses near perihelion to be closer together within the window. But, because the time between successive eclipses in a Saros series is slightly greater than a whole number of years, the alignment with perihelion cannot be maintained for long. Eventually, alignment will be with aphelion, which causes the opposite effect: a greater movement through the window of opportunity and thus a spreading out of the eclipses. The overall effect will thus be to create "bunches" of eclipses around the perihelion position.
The distance between these bunches is [currently] small enough that, although there will usually only be two bunches within the window at the same time, it is just possible for there to be three. Saros series with three bunches will have more eclipses than those with two, but will be in the minority. It is not possible to have a series with an "intermediate" number of eclipses because the extent of a bunch is smaller than the amount a bunch moves from series to series: a bunch will thus either be entirely within the window or not in it at all.
Over time, the shift in position from series to series gets steadily larger due to small changes in the Moon's orbital periods. This increases the distance between the bunches and so, when the distance just exceeds half the window width, it will no longer be possible to fit three bunches within the window. When this happens, the number of eclipses in a series will be fairly constant as eclipses leave the window at the same rate as they enter.
Further increases in the shift will result in it sometimes being the case that there is only one bunch within the window, as a new bunch cannot enter until a short time after an old one has left. This will cause some series to have a much smaller number of eclipses, in the same way that having three bunches instead of two resulted in a much larger total. This process will take a very long time to result in "one bunch in the window" being the norm, however, as this would require the distance between bunches to equal the window width i.e. the distance between bunches would have to almost double. Another effect takes over well before this point: the reduction in the eccentricity of the Earth's orbit.
The eccentricity is currently slowly decreasing, heading for an historic low point in about 30,000yrs time. Because the magnitude of the aphelion/perihelion effect, and thus the extent of the "bunching" discussed above, is determined by the eccentricity, a steady decrease implies that any bunches will be much less tightly packed in the far future. How many bunches there are within the eclipse window thus becomes less important, as their presence or absence will have less and less effect on total numbers. In the end, eclipses will be fairly evenly spread throughout the window and so the number in each series will be about the same. The actual number will now be much smaller, however, because the continual increase in the amount of movement through the window will result in eclipses within the window being further apart and thus fewer in number. Although the eccentricity will eventually start to increase, the greater amount of bunching this will cause will have little effect on overall numbers due to the very large spacing between eclipses by then.
Looking in the opposite direction, the eccentricity reached a peak in about 10,000BC before decreasing again at still earlier times, resulting in a slight additional increase in numbers per series around this time as the bunching of eclipses would have been a little tighter then. This effect is secondary to a more dramatic effect, however, due to the way changes in the eccentricity affect the Moon's orbital motion. This causes the number of eclipses per series to reflect the peak in the eccentricity, resulting in a slow rise to a maximum value followed by an equally slow fall-off as we go further back in time.
Restricting ourselves to the more straightforward era shown by Fig.13, however, the graph of number of eclipses per series should start with regular but well-spaced peaks above a baseline, will move on to a constant value as the peaks disappear and will then show troughs as numbers periodically but increasingly dip below the baseline. Ultimately, these troughs will also disappear as the baseline continues the steady descent it has made right from the beginning, caused by the ever-increasing distance between eclipses in the window of opportunity. Just like Fig.13 does, in fact!
However, this simple picture is considerably confused by effects caused by the eccentric orbit of the Moon. Firstly, because an Inex interval is equal to a whole number of anomalistic (perigee-to-perigee) lunar orbits plus two-thirds, the full-Moon and apogee/perigee states of the Moon are only "in step" once every three Inex periods. This results in there being three distinct, and often quite different, sets of Saros series phenomena existing at the same time rather than just one. Secondly, because the extent of the shift through the window is influenced by the apogee/perigee situation (though to a much smaller extent than the aphelion/perihelion position), series can gain or, more commonly, lose numbers of eclipses relative to their neighbours which can result in the disappearance of expected peaks in the graph of number of eclipses per series.
Finally, because of the requirement that each bunch of eclipses must be a whole number of perihelion-to-perihelion intervals apart (as bunching can only occur at perihelion), the periodicity of the whole process is quite tightly constrained. Currently, it is around 19 series but, due to the slow changes in the Moon's orbit over time already mentioned, it has been about 22 series in the distant past and will slowly decrease to about 15 series in the far future.
So there you have it. To return to the quotation from Meeus with which I opened, "We fear, however, that there is no simple explanation for these variations in the composition of the successive Saros series." Having read what I have laid out in this article, you may well agree!